Every once in a while it is worth reviewing the basic physics behind the greenhouse effect and global warming. Sometimes all the debate about global warming in the media loses focus of the fact that the world really is governed by the laws of physics. Unfortunately, many internet explanations get dumbed down to the point of having an atmosphere that serves as a single “slab” between the ground and space, and has a bunch of colorful arrows coming out of it and bouncing off it, etc. This is a useless explanation, and gives no justice to understanding what is happening. Two encounters in the outside world recently prompted me to do another post just to have a reference handy, and I’m using this to replace an older post which I entitled “just a few more molecules.” There’s also been an interesting episode with Dr. Andy Lacis from NASA GISS over at Dot Earth which I’d like to elaborate on.
We begin with the Planck function, which describes the radiation emitted from a blackbody at a specified wavelength and temperature:
This has physical dimensions of intensity (power per unit area per unit solid angle) per unit wavelength, often in W m-2 µm-1 steradian-1 (a steradian is essentially the 3-D analog of what angles are in two-space; there are 4π steradians in a sphere). h, c and k are constants, λ is the wavelength, and T is the temperature. An important note is that dB/dT > 0 for all wavelengths, which suggests that increasing the temperature increases the intensity at all wavelengths.
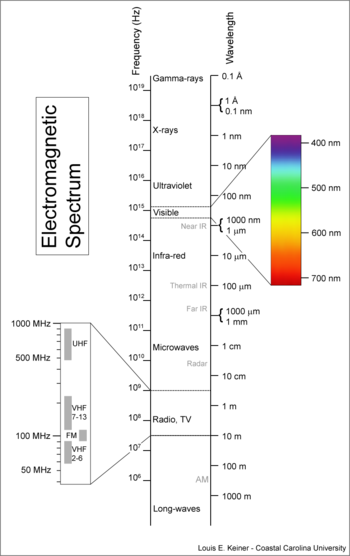
For review, the electromagnetic spectrum is presented below
For our purposes, we’re mostly interested in the fact that the Earth receives energy in the shorter wavelength portions of the spectrum, while it loses its energy in the infrared portions of the spectrum. This distinction between incoming and outgoing energy is made easy for planets such as Earth, where there is essentially no overlap and the two can be treated independently. This would not be the case on very hot planets that could radiate at some several thousand degrees K.
It can be difficult to interpret the Planck law simply by looking at the formula, so it is worth plotting the Planck function to visualize.

http://physics.schooltool.nl/irspectroscopy/images/planck_black-body_radiation.png
As we’ve established, the first thing to note is that the total area under the curve increases as temperature increases, corresponding to increased emission at each wavelength and in total as the object becomes hotter. We can also see that the peak wavelength shifts to shorter values as temperature increases. The wavelength of maximum emission can be obtained by setting and solving for the wavelength, which gives Wien’s law (~ 2897 µm K / T). The Planck law thus helps us understand why the Earth emits primarily in the longwave, infrared portion of the spectrum, while the sun emits primarily in shorter wavelengths, much of which is in the visible and near-infrared portion of the spectrum.
We can integrate the Planck function over all wavelengths to get the total power output per unit area, which gives us the Stefan-Boltzmann law. We also include a factor of to account for the solid angle integration over a hemisphere.
where is the Stefan-Boltzmann constant, approx. 5.670 x 10-8 W m-2K-4.
We’re now in a position to formulate the most basic radiative balance equation for planets. We can assume the no-atmosphere Earth radiates like a blackbody, although non-perfect radiators emit like where
represents the ratio of how well the emitter is to a perfect blackbody. At equilibrium, this radiative balance is:
where is the ratio of the surface area of the planet to the cross-section (which is very close to 4 for planets), (1-
) is the fraction of absorbed solar radiation (since
is the albedo=reflectivity) that comes from the solar constant S0, and R is the distance to the star in astronomical units. It automatically takes on a value of one for the Earth-sun mean distance and S0 takes on a value of 1370 W m-2. This radiative balance equation states that, at equilibrium, the Earth wants to lose as much infrared radiation to space as it absorbs by the sun. This basic calculation assumes no atmosphere and a uniform planetary temperature. This uniform temperature assumption is nearly valid for planets such as Earth or Venus, but is way off the mark for those which exhibit extremely strong diurnal gradients like Mercury or the moon.
Present Earth albedo is 0.3, so the calculated equilibrium temperature for our planet would be 255 K. This is well below the freezing point of 273.15 K. This planet would be frozen down to the tropics! Since the sun is the only important incoming source of energy to Earth, internal heating plays a negligible role in the planetary energy balance (although is important for gaseous planets like Jupiter), then we can say that the difference between the observed 288 K and 255 calculated temperature is due to the fact that the atmosphere acts to inhibit the efficiency by which outgoing infrared radiation escapes to space. In radiative terms, this is:
This post is not meant to discuss why greenhouse gases are greenhouse gases. But it should be noted that the bulk of the atmosphere, including N2, O2, and Argon are not infrared active molecules. It is actually very common, although perhaps not well known, for diatomic molecules to become good greenhouse gases in pressure-induced planetary situations (this is important on Jupiter for instance, and on the dense atmosphere of Titan), but aside from their role in broadening the lines of the other greenhouse gas molecules, they play essentially no role in Earth’s radiation balance. The absorbing gases discussed in this post include water vapor, clouds (which aren’t gases, but still contribute to the greenhouse effect), CO2, methane, N2O, ozone, and a variety of other lesser important constituents.
The rest of this post is basically a description of the manner in which absorption by these molecules affect the planetary energy budget. Before that happens, a quick review of the temperature structure of the atmosphere. It can be shown quite easily that the temperature of the atmosphere drops with height. For air devoid of water vapor, this rate is . For a moist atmosphere, this rate is reduced to about -6.5 K km-1 due to latent heat release of condensation, which results in the temperature still decreasing, but less rapidly than that rate given by the dry adiabat. The real atmosphere at a given time is generally somewhere between a dry and moist adiabat.
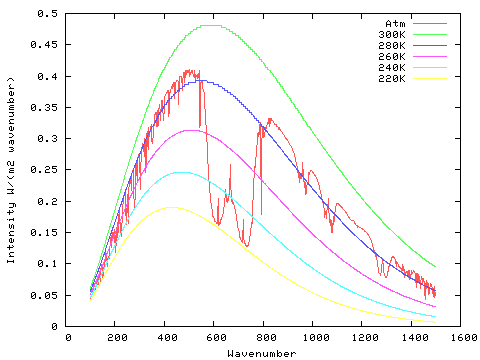
We now have the tools to look at some model emission outputs. As I’ve done before, I’ll use the MODTRAN model available from Prof. David Archer’s web page.
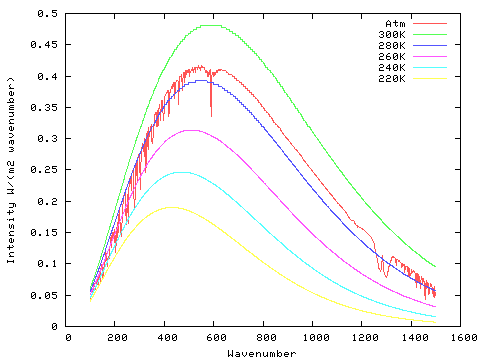
The settings I will use in all experiments is to keep the sensor at 70 km looking down, U.S. 1972 Standard Atmosphere, and for the sake of instruction, no clouds, water vapor, or rain. Let’s begin by constructing a plot in which CO2, CH4, and ozone are all set to zero. We obtain:
What the plot shows is a set of colored line which correspond to blackbody curves at specified temperatures. Note that we’re now looking at wavenumber on the x-axis, which is the inverse of wavelength, so the peak on the graph now shifts to the right for increasing temperature. 10,000 divided by microns will give you wavenumber in inverse centimeters, which are common units used in this case.
The red squiggly line corresponds to the Earth curve with the specified settings. The model is fixed at 288.20 K unless changed in the input settings, so the red curve is somewhere between the 280 and 300 K blackbody curves, though not emitting perfectly like a blackbody. We see that Iout is 346.97 W m-2. Now let’s add just 2 parts per million of CO2 into the atmosphere.
It is useful in atmospheric radiation to describe an optical thickness (or optical depth), along a vertical path,
where k is an absorption coefficient, and is the density of absorber. The transmittance through an absorbing medium goes like
.
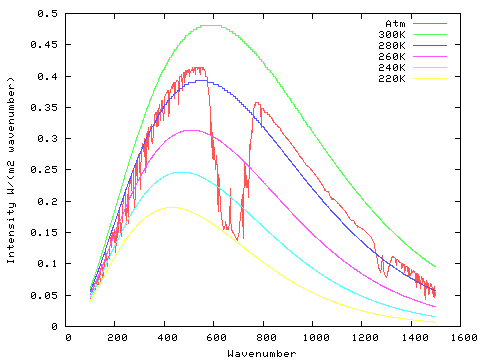
The noticeable difference in the second figure is that there is now a “bite” taken out of the Outgoing Longwave Radiation (OLR) curve. Viewed from space, an observer wearing infrared goggles would now see emission emanating from colder layers of the atmosphere. In parts of the spectrum where the atmosphere is optically thick, the radiation to space occurs at the temperature of the high, cold parts of the atmosphere. On the other hand, the transmitted ground emission will dominate the OLR when the atmosphere is fairly transparent.
In the 2 ppm case, the OLR is now 338.8 W m-2. Since the area under the curve has been reduced due to the blip caused by CO2, we are now interested in re-establishing the outgoing flux to be 346.97 W m-2. We can do this by raising the temperature in the model by 1.9 C. Simply through fundamental physics, it can be shown that the temperature must increase as greenhouse gases are added to the atmosphere. Now let’s re-set the ground T offset to zero and put in 50 ppm of CO2 into the model. The resulting graph is:
If the greenhouse gas in question were absorbing only in this limited interval, then increasing its concentration further could not bring down the OLR any further, since in the spectral region where the gas is radiatively active, the atmosphere is already radiating at the coldest temperature possible. Technically, since the stratosphere cools with more greenhouse gases, this would have a minor effect on the OLR, but it is a negligible one for our purposes. If we change the amount of CO2, the intensity of light in this range does not get any lower. This is called band saturation.
Instead, further increasing CO2 will decrease the OLR essentially by filling the “wings” of the spectral bands where the atmosphere is optically thin. We can see this by putting in a modern concentration of 390 ppm CO2. The manner in which the “wings” of spectral lines become important is discussed in Dr. Ray Pierrehumbert’s RealClimate post on Angstrom.
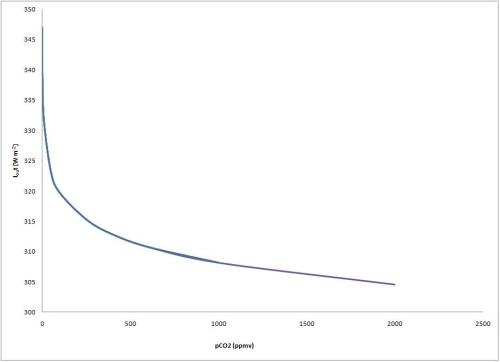
Greenhouse gases have absorption whose overall strength decays rapidly with distance in wavenumber from the central peak, which helps explain the reason in which the OLR change goes like the logarithm of CO2 amount at concentrations relevant to the global warming problem. Here is a plot of the outgoing energy term as a function of CO2 concentration. This is a visual confirmation that after you have already introduced some CO2 into the atmosphere and are up to realistic concentrations, every doubling will produce the same radiative forcing.
One could compute the temperature change for a perturbation in the OLR for just a change in CO2, leaving other climate variables constant.
which gives a ~1 C rise in temperature for a 4 W m-2 radiative forcing. This would be the temperature response to a CO2 doubling if only the Planck radiative feedback were important. Unfortunately, life is not that easy, and you need to figure in the feedbacks from water vapor, clouds, albedo, etc.
Now let’s put some crazy amounts of CO2 into the model, say 10,000 ppm.
As we can see, the bite in the spectrum is not getting any deeper, but it is getting considerably wider. This is very important for the greenhouse effect, since you will essentially never become “saturated” and thus you will keep getting warming with more and more CO2. We should now be in a position to interpret a plot of atmosphere transmission or absorption,
This helps to visualize the percent absorption by atmospheric constituents on a scale from 0 to 1 as a function of wavelength. It is important to note that the absorption coefficient is a strong function of wavelength. Although radiative transfer equations would often be much easier to solve if the optical thickness was independent on wavelength (the so-called grey gas approximation), alas, this is not how life operates. This is somewhat convenient though, since if the atmosphere behaved like a grey gas, changing CO2 from a “nice concentration” just a tiny bit would make the planet virtually inhabitable, setting an extremely narrow window for proper atmospheric conditions suitable for life.
Let’s go back to our model outputs obtained from MODTRAN. Note that there is a small “blip” at the bottom of the center CO2 band, which becomes very apparent in the 10,000 ppm case. This is a signature of the stratosphere, where temperature increases with height. You get this blip in a very high CO2 case since the atmosphere is so absorbing in the center of the band that emission is coming from above the tropopause, while in the wings, where absorption is weaker, emission comes from below. This “blip” is potentially useful to planetary scientists looking for life on distant planets, since a hot stratosphere could be a signature for ozone, and thus oxygen. This blip would probably not be real if you were to really add that much CO2, since the stratosphere cools with more CO2 as it becomes a less effective absorber but a better emitter of radiation. You can somewhat see this if you lower the sensor altitude to 30 km or so.
It is really important (in fact, essential) to understand that the greenhouse effect requires colder air aloft to work with, as you essentially replace strong surface radiation with weaker emission from higher layers. This is why adding CO2 creates an energy imbalance at the top-of-atmosphere. In an isothermal atmosphere, you could not get a greenhouse effect.
The effective height “H” above is obviously dependent on wavelength, and moves higher as the opacity in increased in that region.
So…review: Because of energy balance, the planet must get rid to space as much energy as it receives from the sun. Averaged over the Earth, taking into account the albedo and geometry, this is about 240 W m-2. In the absence of an atmosphere, this flux of radiation is lost by the surface by . With an atmosphere, this flux of radiation is allowed to emanate from upper, colder layers of the atmosphere, say on average at some altitude H. Increasing greenhouse gases increases the altitude of H, a height in the atmosphere which depends on wavelength, and characterizes a level of mean emission to space. Because the atmosphere is now emitting from colder levels of the atmosphere, the OLR has decreased, and the result is that the planet must warm to re-establish radiative equilibrium.
Radiative transfer in the earth’s atmosphere is not particularly amenable to simple formulas because the atmosphere is semi-transparent to differing degree at different wavelengths. For example, there is a popular formula for a 2-layer equilibrium relationship which is obtained by treating the atmosphere as a slab and solving multiple equations for the energy balance of each slab. This states that Ts = 21/4Teff (or generalizing, Ts = (n+1)1/4Teff, where n is the number of layers). It can be found, for example, in David Archer’s “Understanding the Forecast”, or Dennis Hartmann’s “Global Physical Climatology.” As an exercise, I urge readers unfamiliar with this model to try to derive this expression using principles discussed here. You can also refer to these lecture notes (slide 16, 17 on radiative-convective equilibrium) if you need help. This is valid for an isothermal grey absorbing layer above a Planck emitting ground, and applies only when the layers are either totally transparent or totally opaque. Such a model can be made more complex by allowing for non-unity absorptivity/emissivities in different layers, allowing for shortwave absorption in the atmosphere, etc. These lend very good insight into the nature of temperature inversions, geo-engineering, how one might need to later the albedo to offset CO2 increase, etc. One might find out for instance that if we perfectly offset enhanced absorptivity by increased reflectivity to keep the surface temperature constant, you can still get a change in atmospheric temperatures, which would thus have implications for the lapse rate and stability. So, I urge experiments with these approaches. Although this sets the stage for basic textbook explanations to get a feel for radiative balance, in GCM’s, the radiative transfer problem needs to be addressed numerically, with a sufficient number of vertical layers to resolve the atmospheric temperature and absorber distribution, with an acceptable amount of spectral intervals to resolve the spectral dependence of the contributing gases, and account overlap between various atmospheric constituents. Further, using a 2-layer model in a fully transparent shortwave and fully opaque longwave atmosphere achieves a surface temperature of 303 K, which introduces another obvious problem with this model, notably the lack of convection which removes heat from the surface, and also establishes the vertical temperature profile of the atmosphere from which the greenhouse effect relies on.
Saturation
In modern concentrations, every doubling of CO2 will reduce the OLR by about 4 W m-2. This doesn’t hold at very low concentrations as we’ve seen, but also at very high concentrations. The phenomena of band saturation also allows us to say something interesting when comparing two greenhouse gases side-by-side.
It’s often noted that methane is “20x more powerful than CO2” (see a quick google result for proof). This statement can potentially be misleading, so it is worth clarifying just what it means.
The natural 33 K greenhouse effect has a much larger influence from CO2 than it does CH4. Even in the context of how the greenhouse effect is changing, CO2 is currently a much stronger forcing agent than CH4. In what sense is CH4 more powerful? This is only if we compare CO2 and CH4 side-by-side and allow the two gases to change by some incremental amount at existing background concentrations. It is only because CH4 is far less abundant in the atmosphere that adding, say, 1 ppm of CH4 will produce a larger radiative forcing than would be adding 1 ppm of CO2 to today’s atmosphere. This has nothing to do with any intrinsic property of the gas. If CO2 were far less abundant, and CH4 much more abundant, then adding a certain about of CO2 would be more effective at reducing the OLR, and we would then say “CO2 is a more powerful greenhouse gas.”
“The most important greenhouse gas”
Dr. Andy Lacis of NASA GISS is a radiative transfer expert and has worked on planetary climate stuff for a long time now. He has recently been hailed by global warming skeptics for a criticism of an early draft of the IPCC summary for policy makers. Lacis had plenty of words to say to the “skeptics” about this at Dot Earth (linked at the top of the post, you can follow the several posts about it) but finally Lacis felt the need to simply go back and review the basic physics of radiative balance which constrains the global climate. Along the way, Lacis is quoted as saying
“The bottom line is that CO2 is absolutely, positively, and without question, the single most important greenhouse gas in the atmosphere. It acts very much like a control knob that determines the overall strength of the Earth’s greenhouse effect.”
This generated some interesting comments, but let’s examine what Dr. Lacis was getting at, since I certainly agree with this.
If you break down the natural greenhouse effect into a fractional contribution between constituents you end up getting that about 50% of the 33 K greenhouse effect is due to water vapor, about 25% to clouds, 20% to CO2, and the remaining 5% to the other non-condensable greenhouse gases such as ozone, methane, nitrous oxide, and so forth. It is therefore popularly noted, from popular discussion to textbooks to academic papers that “water vapor is the most important greenhouse gas” in the atmosphere. While it is true that water vapor represents the largest source of infrared opacity in the atmosphere, the claim that it is “most important” is just sloppy terminology with no real attempt to define what exactly that is supposed to mean. At some level, this could just be academic disagreement, but it is worth putting into perspective that CO2 is indeed the control knob which governs the global climate.
The non-condensable greenhouse gases (e.g., CO2, CH4, etc) are those which do not precipitate from the atmosphere at Earth-like conditions. They can support a temperature of nearly 10 K above that which is determined by equilibrium with the incoming solar radiation on their own. Water vapor, on the other hand, is controlled by temperature. I have a rather long post discussing the radiative feedbacks which are important for understanding the sensitivity of climate, which defines the temperature response per unit radiative forcing. The amount of water vapor in the atmosphere is not set by sources and sinks, but has an upper limit on its concentration until it precipitates out. Because of this, the non-condensable greenhouse gases act as the backbone upon which water vapor and clouds can really do their stuff. Without them, you would also get a near collapse of the terrestrial greenhouse effect as water vapor content declined nearly exponentially with a declining temperature. This scenario would cause a runaway snowball Earth and a much higher surface albedo. Indeed, removing either water vapor or CO2 from the atmosphere would trigger glaciation down to the tropics. When thinking about the evolution of Earth’s climate though, it is CO2 which changes, and water vapor which is dragged along to amplify the total response.





Very nicely done. You’ve covered a lot of ground with this post. What stands out about this is that you don’t cut corners, but have given the real physics of the atmospheric greenhouse effect without dumbing it down. Kudos. This deserves to be a widely known reference for use in internet discussions.
Yes, I agree with sylas. Very clearly done. Whenever I talk to sceptics (which, unfortunately is rather too often) I eventually go back to the physics. The rate and pattern of temperature rise following increased carbon dioxide is irrelevant…eventually the T MUST increase. Even the clever sceptics like Steve McIntyre accept that I think. They (cleverly) only discuss the trees, not the wood! If only we could educate everybody else, then there would be a political mandate for action. At the moment though it has stalled and there’s not much sign of it moving soon.
Thank you. The point where you said “it can be shown that the temperature must increase as greenhouse gases are added to the atmosphere” marks another significant step in my slow learning of these concepts. I’ll be studying this post and its related links for awhile and perhaps I’ll be able to articulate a few vague questions I still have. (By the way, I’m guilty of many simplified slabs and colorful arrows on this subject).
jg
Excellent post!
Much appreciated; especially the progressive model emission graphs. They are very helpful in visualizing changing levels of CO2.
A few comments…
1) In the Saturation paragraph, the following is stated:
“In modern concentrations, every doubling of CO2 will reduce the OLR by about 4 W m-2. This doesn’t hold at very low concentrations as we’ve seen, but also at very high concentrations.”
For the initial 2ppm CO2 case, OLR was reduced from 346.97 to 338.8.
So, 2 ppm CO2 was worth about 8.2 W m-2 or about 4.1 W m-2/ppm.
Understand that it would have been more work, but it may have been helpful to some readers if an OLR calculation were made at 4ppm CO2 to illustrate the magnitude of warming from doubling at low concentrations.
2) “This blip would probably not be real if you were to really add that much CO2, since the stratosphere cools with more CO2, since it becomes a less effective absorber but a better emitter of radiation.”
I don’t understand this sentence and it may be a run on. It would probably be easier to understand if it were broken up or re-worded.
3) “This is somewhat convenient though, since if the atmosphere behaved like a grey gas, changing CO2 from a “nice concentration” just a tiny bit would make the planet virtually inhabitable, setting an extremely narrow window for proper atmospheric conditions suitable for life.”
I’m struggling to wrap my mind around this concept. Could you elaborate on this some.
Thanks again!
@Andrew,
“This blip would probably not be real if you were to really add that much CO2, since the stratosphere cools with more CO2, since it becomes a less effective absorber but a better emitter of radiation.”
I don’t understand this sentence and it may be a run on. It would probably be easier to understand if it were broken up or re-worded.”
Understanding the stratosphere is generally problematic for laypeople (and it’s difficult to explain it without making some mistakes and/or misrepresentations)
I’ll try my best to explain it without making serious mistakes or getting too technical:
– The stratosphere is optically thin so it’s a poor absorber of thermal radiation.
– The stratosphere is very dry.
– The stratosphere is warmed mostly by UV radiation. This explains the cooling of the stratosphere caused by ozone depletion.
– Greenhouse gases not only absorb thermal radiation, they also emit it.
So, if you were to analyze the energy budget of the stratosphere you’d see that adding GHGs would increase its ability to radiate heat away. The only way for the stratosphere to warm is to capture more UV radiation and loose very little thermal radiation.
Hope I was clear enough.
Lucas;
Thanks for trying to help. It maybe the grammar that I’m struggling with:
“This blip would probably not be real if you were to really add that much CO2, since the stratosphere cools with more CO2, since it becomes a less effective absorber but a better emitter of radiation.”
Could this be re-worded as follows and still correct:
“This blip would probably not be real if you were to really add that much CO2 since the stratosphere cools with more CO2 (it becomes a less effective absorber) and becomes a better emitter of radiation at the same time.”
Response: I tried changing a bit. Hope Lucas’ comment makes sense. A more complete explanation involves the spectral dependence, as the stratospheric emission has to be in a limited wavelength band if you’re going to get cooling while still respecting the planetary radiation balance. The dynamic changes in the grey atmosphere case. Specifically, with more CO2, the stratosphere becomes shielded more strongly from upwelling radiation from below by the increased opacity in the 15 micron region, while it continues to emit effectively in those regions, leading to strong cooling. The stratosphere also cools with ozone loss as well– chris
That’s much clearer, so to speak:
Lemme whack at it if I may:
> with more CO2, the stratosphere becomes shielded more …
> from upwelling radiation
(more CO2 below intercepts more outgoing infrared), while
the increase in the CO2 in the stratosphere
> continues to emit effectively, leading to strong cooling
> of the stratosphere and more warming below the stratosphere.
Why then do we not get a clear cut progressive increase in the rate of temperature rise as the tipping point predicted by the climate scientists demands? The lack of it proves that no mattter how plausible the scientific theory is, it is unsound in reality.
Response: There is no inconsistency between observed surface temperatures and predictions. And we’re talking about real predictions, not the phantom ones. Sorry.– chris
D Cage – the phrase ‘tipping point’ might be intended to refer to either crossing (unmeasured possible) thresholds at which strong positive biogeochemical feedbacks might kick in (which is distinct from those feedbacks considered in Charney climate sensitivity to radiative forcing), or it might be intended to refer to irreversible changes (extinctions) or changes that occur that take a long time to reverse even if forcing is quickly retracted toward preindustrial values.
D Cage–
Do you ever wonder where you get your ideas and why you believe them reliable?
Here’s one way to check this kind of thing:
http://www.google.com/search?q=climate+co2+%22clear+cut+progressive+increase+in+the+rate+of+temperature+rise%22
— if Google is right, literally no one on Earth has ever said that, except you.
(Which raises the question, where did you get the idea — it’s certainly a familiar mistaken idea you’ll find on PR blogs, but it’s not ever said by scientists — you can check that with Google Scholar):
http://scholar.google.com/scholar?q=climate+co2+%22clear+cut+progressive+increase+in+the+rate+of+temperature+rise%22
No offense intended — I’m an amateur reader, I know how hard this is to learn and how easy it is to get wrong information stuck in one’s head, and “it’s a poor memory that only works backward” and all of us tend to think we know stuff nobody ever told us. Checking our own ideas is a good place to begin asking questions. Google can help.
Why then do we not get a clear cut progressive increase in the rate of temperature rise as the tipping point predicted by the climate scientists demands?
It’s not at all clear why you think anything climate scientists say implies a clear cut progressive increase in the rate of temperature rise, but it doesn’t.
The lack of it proves that no mattter how plausible the scientific theory is, it is unsound in reality.
Shouldn’t you at least wait for an answer to your question before coming to that conclusion? And just what exactly is it that is “unsound in reality”? If in fact the maximum average global temperature increases over time, even if there isn’t ” clear cut progressive increase in the rate of temperature rise”, then global warming is “sound in reality”.
In my opinion the model described in the article is only a half truth:
– The Earth is described as a hemisphere, but in reality it is a sphere, and there is always the other hemisphere which is not getting radiation from the sun.
– Water is not only a greenhouse gas, but has other vitally important functions: It carries heat up to higher levels in the atmosphere where the heat energy is released when water condenses. And clouds have a strong albedo effect.
Response: For the first point, I was not talking about the incoming solar energy or the day-night cycle. I was talking about integrating over all solid angles which are of relevance to us, since emission is essentially independent of angle (isotropic). This is the way to express radiation from the surface of the Earth. I’m not talking about “northern” or “southern” hemispheres as we’ve come to call them or the day-side or night-side of the planet. I should maybe have described this point in more detail, but describing the mathematical justification behind the factor of pi in the flux density expression would have gotten us a little sidetracked from the point of the post.
As for your second point, the planet itself is only gaining and losing heat by radiation, since it is surrounded by a vacuum. You are not describing a component of the planetary energy budget, but rather of the surface energy budget. Indeed, if the surface were only losing heat by radiation, the 33 K enhancement would be a substantial underestimate of the strength of the greenhouse effect. The mode of heat transport you describe is understood and has been included even in simple models going back to at least Manabe in the 60’s– chris
I agree with sylas. It’s about time somebody put out a description for the layman that didn’t cut corners. Most cartoon illustrations totally gloss over this important point: “In an isothermal atmosphere, you could not get a greenhouse effect.”
Yup, so far so good. Perhaps, as you imply, a more comprehensive mathematical treatment of the whole system would be even more convincing, including how the greenhouse effect affects winds, cloud formation on different latitudes, possible changes in the prevalence of different cloud types, and the distribution of thermal energy over the latitudes.
Response: You are simply throwing up smokescreens now. My blog is not a textbook; essentially any book on atmospheric radiation will discuss solid angles in detail. I suggest Grant Petty’s “Introduction to Atmospheric Radiation” for one of the more readable approaches. My blog is also not a coupled global climate model. It is simply a description which allows the reader to get an intuitive feel for how the greenhouse effect works. — chris
OK, I understand.
Thank you for the discussion.
I = Intensity (flux per unit area per unit solid angle, wherein the unit area is perpendicular to the direction considered)
F = flux per unit area (in casual conversation, this is often referred to as ‘flux’, but that is technically not accurate).
I and F can also be considered per unit spectrum (wavelength or frequency) at a given part of the spectrum).
w = a solid angle
dF = contribution to F of intensity in all directions within solid angle dw
dF = cos(q)* I * dw
where q is the angle from perpendicular to the unit area that F goes through; the factor cos(q) accounts for the reduced contribution to F at or through a unit area from an I*dw that comes from a slanted direction.
q is one of the spherical coordinates – for a horizontal surface, for radiation downward through a unit horizontal area, q is the zenith angle (for upward radiation, q would by the angle from nadir (opposite direction from zenith).
Use L for azimuthal* angle (q is analogous to a colatitude: cos(q) = sin(latitude) when north pole is zenith, and L is than analogous to longitude)
dw in the direction q,L:
dw = sin(q)*dq*dL
the factor sin(q) is required because, projected onto a unit sphere (radius 1), the same dL*dq is a smaller area for small q.
(note solid angle, measured in steradians, = surface area of the projection of the solid angle onto a unit sphere from the center. Note that a ‘regular’ angle, measured in radians, = arc length of the projection of the angle onto a circle from the center.)
Integral of dw over full circle of L:
dw = 2*pi * sin(q) * dq
notice this is a small angle dq multiplied the circumference of a circle (the length of a line of colatitude).
Integral of dw over hemisphere (q = 0 to q = pi/2):
dw = 2*pi*[ -cos(pi/2) – -cos(0) ] = 2*pi*(0 + 1) = 2*pi
which is half the surface area of a unit sphere!
Integral of dF over a hemisphere for isotropic radiation intensity I (isotropic radiation means that I is constant over q,L; perfect blackbodies emit isotropic, unpolarized, incoherent radiation).
dF
= I * cos(q)*dw
= I * cos(q)*sin(q) * dL * dq
= I * (1/2)*sin(2*q) * dL * dq
integral over full circle of L:
dF
= 2*pi * I * cos(q)*sin(q) * dq
= 2*pi * I * (1/2)*sin(2*q) * dq
(this is why F = pi*I, not 2pi*I, for isotropic I)
= pi * I * sin(2*q) * dq
Integral from q=0 to q=pi/2 (the F from all radiation at/from or through the unit area from/to one side of that unit area)
F
= pi * I * (1/2) * [-cos(pi) – -cos(0) ]
= pi * I * (1/2) * 2
= pi * I
Why consider only a hemisphere?
That is relevant to finding the flux upward or downward. The net upward flux can be found either by integrating I over the whole sphere, subtracting the downward flux from the upward flux, or integrating net I over one hemisphere, where net I is forward I minus I from the opposite direction for each direction. These are all mathematically equivalent.
A net F requires some anisotropy – that is, I must not be constant over direction. I could be constant over each of two hemispheres and different between them, or it could vary continuously, or in some more complex way. The later is the case for radiation through the atmosphere.
For LW (longwave) radiation (the radiation at wavelengths where the blackbody I is significant for typical surface and atmospheric temperatures (excluding the thermosphere, which is optically very very very thin), this is because I at near horizontal directions must come from paths that are much longer over thin nearly isothermal (typically) layers and thus tends to be near the blackbody I for the temperature at that location (except when/where opacity is so small that the layer is significantly transparent over distances over which temperature varies signficantly horizontally or over which the curve of the Earth causes the path to diverge from horizontal), whereas nearly vertical paths cross larger temperature variations per unit distance and thus (except when opacity is too high) I at q nearer 0 or nearer pi can diverge a lot from the local blackbody value). Looking up from the surface, I will tend to be largest near the horizon and smaller near vertical, along paths through which the lower warmer air is optically thinner and thus doesn’t hide the coolness of the upper troposphere and lower stratosphere as much, and where the whole atmosphere doesn’t hide the darkness of space as much – but this varies with wavelength and local temperature and humidity and cloud conditions, and the opposite pattern may be observed under an inversion when there are clouds within the inversion or at wavelengths with greater opacity, etc. Looking down from either space or the tropopause level or within the stratosphere, the largest I tends to be seen looking nearly straigtht down for the same reasons, but with variations from that pattern for the same reasons.
For SW radiation (solar radiation, wavelengths shorter than about ~ 4 microns), I is not constant over the solar disc, varying between sunspots and faculae, etc, and more generally decreasing from the center because the photosphere is not isothermal with depth but cools with height, and directions toward the solar limb cross larger distances through the cooler outer part and thus the visibility of the warmer part is reduced. However, it can be approximated as constant over the solar disc’s solid angle for some purposes. In space, away from any other object, SW drops to near 0 in nearly all directions outside the solar disc’s solid angle, with some small contribution from the corona, etc, and very small solid angles of large I from the stars. Near the Earth, a significant amount of solid angle will have significant I from reflected/scattered radiation. Within the atmosphere, some nonzero I is distributed over the whole sphere (not isotropically – in clear skies the doward scattered I is larger near the horizon due to the longer paths through the air, and very large near the sun due to forward scattering. For an initial beam of radiation, successive scatterings tend to broading photons over a larger range of solid angles and reduce the anisotropy over the sphere, even if most individual scatterings are foward scatterings) due to scattering/reflection, while the I within the solar disc is reduced for the same reason.
This is all for considering radiation at one location on the Earth; it can be averaged over the globe (two hemispheres) of the Earth to find a global average (at a given vertical position).
Thanks for the explanation, but I am having some difficulties, probably because my perspective is wrong.
1. The surface emits energy in 3 ways – direct conduction (minor), net radiation (ie stephan-botlzmann minus the back-radiation from the atmosphere) (minor), and evaporated water (major). All three are manifested in the atmosphere as sensible heat (at different levels – conduction at the surface, radiation in the low atmosphere close to the surface, and latent heat of condensation into the clouds). This is then convected upwards, the heat transport mechanism within the atmosphere.
2. The earth must radiate its received energy in some form into space. This radiation must come from:
A. The surface – as you point out, the non-absorbed portion of the surface radiation, 15-30% of the 390W/m^2, belts off into space, with a characteristic emission temperature of 288 DegC or so.
B. Gases in the atmosphere which are capable of emitting photons in the infra-red. The candidates are Ozone, H2O and CO2. These all emit at different levels in the atmosphere. The top of the water vapour is at the lowest level and is therefore hotter. Water Vapour is also capable of emitting in far more of the spectrum than the other two gases (see your absorption spectrum). So the majority of emissions from most of the planet must be coming from the top of the water vapour, ie around 5000m and -10DegC. The next gas is CO2. For the 15um band (the most fierce emission frequency) the top of the CO2 is really high – somewhere in the tropopause/stratosphere. This is quite cold, around -50DegC.
3. I would therefore expect to see the following in an emission spectrum from Earth:
A. Strong emission (characteristic temperature about 288 DegK) in the non-absorbed bands.
B. Medium emission (characteristic temperatures ranging from 288 down to about 260 DegK, depending on wavelength) in the water bands. This would vary enormously with latitude and landform.
C. Weak emission (characteristic temperatures around 220 DegK) in the CO2 bands.
D. Somewhat stronger emission in the Ozone bands, as the temperature is a little higher.
4. I don’t see this in your diagrams.
5. Because the emissions from CO2 are at so high an altitude, the temperature gradient is no longer negative, but is neutral or positive. Varying the optical depth of the CO2 will have no effect on the temperature of the emission, or its power. So there is no major greenhouse effect (energy budget imbalance) from this source.
Re 1 –
a little clarification:
Both radiation and convection are important in transporting heat through the atmosphere.
The surface generally (global time average) loses heat to the atmosphere and space via LW radiation, and generally (global time average) loses heat to the atmosphere via ‘convection’. In this context, the term convection includes the conduction of sensible heat and molecular diffusion of latent heat via water vapor from the surface; these processes are only important for maybe 1 mm or so of air next to the surface as convection occurs much more easily away from the surface (the surface is an impediment to motion, hence the importance of conduction and diffusion next to it). Because of the necessity and dominance of conduction and diffusion immediately next to the surface, and because of the small-scale nature of convective motions near the surface, net radiative heating of the surface can and does sometimes sustain a superadiabatic lapse rate near the surface. Above this, in such conditions where mixing is driven by heating, and also when wind supplies energy to mix the air even without surface heating
…
(sufficient vertical wind shear can overcome stable stratification and mix the air, which interestingly results in a stronger stratification at the top of such a mixed layer since the top of such a layer is cooled and the bottom of such a layer is warmed by the process and whole layer is warmed by the kinetic energy input required to ‘break-up’ the stratification; my understanding* is that kinetic energy generated by differential-heating driven convection can also do the same thing and entrain warmer air (as measured by potential temperature; potential temperature is conserved along a dry adiabat) above the mixed layer to make the mixed layer thicker and perhaps produce an inversion at the top of the mixed layer (*perhaps analogous to the thermocline below the mixed layer of the ocean (?), which is mixed by wind energy and also by differential heating and salinity variations (water is more transparent to some portion of SW wavelengths than it is to LW radiation, so there is some solar heating that takes place beneath the thin surface layer that has net LW cooling (typically), thus solar heating can drive some convection in the upper ocean; also, evaporation at the surface produces cooler,saltier, denser water that can sink; precipitation has the opposite effect on salinity.) (Without the kinetic energy produced by thermally-driven convection (or from the wind), surface heating would produce a mixed layer up to the level where the convective lapse rate from the surface intersects the preexisting lapse rate of the air, and no farther.)
(Kinetic energy is produced from heat energy by thermally-direct convection, with warmer air rising and colder air sinking (at a given pressure level, setting aside variations in composition which are generally of minor importance to atmospheric motions); kinetic energy is converted back to heat energy by thermally-indirect motions (colder air rising, warmer air sinking) and by viscosity (molecular viscosity and mixing of momentum). (Same thing in the ocean; replace temperature with density to account for the role of salinity.) The kinetic energy production is small compared to the heating rates, and most kinetic energy produced within the troposphere is also converted back to heat (at higher entropy than the heating which produced it) within the troposphere or at the surface/within surface material (ocean), and thus the kinetic energy can to a good approximation be neglected in the heat energy budget, but of course kinetic energy is important for atmospheric motions; important thermally-indirect motions above the tropopause level are driven by mechanical energy supplied from below via Rossby waves and gravity waves and various equatorial waves (Rossby, gravity, Rossby-gravity, Kelvin).
…
a mixed layer exists that is dry adiabatic, or (approximately) moist adiabatic when and where the relative humidity is approximately 100 % (there is some complexity in the process of condensation of vapor onto nuclei, etc. – see “Kohler curves”).
(it is possible for the surface to have a net gain from LW radiation from the atmosphere under some conditions, such as under a sufficiently thick and/or cloudy or humid inversion, but this would be unsustainable for a global average given the solar heating of the surface).
In environments where there is deeper convection from the surface, the lapse rate is dry adiabatic below the cloud base, of course.
————–
4. The focus was on the effect of CO2 – for what you are looking for, see Kiehl and Trenberth 1997:
Click to access RadiationBudget.pdf
Your expectations are somewhat correct.
The ozone layer is heated by solar UV but is still not as warm as at the surface, and also, my understanding is that ozone is generally less opaque in its LW absorption band than in the UV wavelengths, so emissions to space at those wavelengthsto space may come from the upper mild stratosphere, but also the lower cooler parts of the stratosphere as well as from the warmer surface and cooler cloud tops when they are present, etc.
Water vapor can reduce the brightness temperature farther than you expect in portions of its spectrum.
5.
Opacity varies over wavelength and this is true within the CO2 absorption band. In the central portion of the band, emission to space will increase with increasing CO2. However, outside the central part of the band, emission will decrease because the opacity is still low enough for much or most radiation to be coming from below the tropopause level. At the tropopause level, there is almost no effect on the net flux within the central part of the band – it is saturated – but farther from the center, there is a decrease in net upward radiation, as radiation from warmer lower levels (surface, lower level CO2, clouds, humidity) is partly blocked and there is an increase in downward radiation from above as the stratosphere radiates more strongly, blocking the darkness of space (I’m not sure if there are any wavelengths at which downard radiation decreases, because the upper warmer stratosphere might never become sufficiently opaque to radiate with a warmer brightness temperature before the lower colder stratosphere is opaque enough to block that radiation from reaching the tropopause).
There is a decrease in net upward LW flux both above the stratosphere and at the tropopause level, with the change at the tropopause level being larger. The difference in the change is a net LW cooling of the stratosphere.
Holding conditions beneath the tropopause steady but allowing temperatures above to reach temporary equilibrium, the stratosphere cools as a result of increased emission to space and decreased radiation from below (with some compensation from increased absorption of radiation from below). After this change, the change (from before the CO2 increase) in net upward LW radiation is larger above the stratosphere and smaller at the tropopause level, because the cooler stratosphere emits less radiation upward and downward. The resulting change in net upward LW flux (change from before the CO2 increase) is now constant from the tropopause upward. This is the tropopause level forcing with stratospheric equilibration, and is a key value of climatic effects. The tropopause level forcing is a heating rate, and heat accumulates below the tropopause level until (accounting for feedbacks) the net upward LW flux increases enough to balance net downward SW radiation at the tropopause level (and so on for other levels, including convection for levels below the tropopause and also regionally, etc.). The stratosphere will also warm up during this process, and this is a positive feedback to tropospheric warming (although an increase in tropopause height actually removes mass from the stratosphere, tending to reduce downward stratospheric emission at the tropopause, but also increasing the opacity of the troposphere and the temperature difference between the surface and tropopause… – my impression is neither of these changes is sizable compared to some other feedbacks like water vapor and lapse rate feedbacks – etc.) – this is why the earlier equilibration was temporary. Stratospheric equilbration is a mathematical process but is actually similar to the sequence of events in reality, because the heat capacity of the stratosphere is quite small and the stratosphere does equilibrate to changes in radiation on timescales shorter than a season (with some important regional deviations from equilibrium due to large-scale overturning patterns).
This pattern of change is similar for greenhouse gas increases in general (including water vapor feedback) with variations in quantities due to variations among spectra and, for water vapor, clouds, and ozone, spatial variation (the increased total emission to space as measured at the top of the atmosphere, such as occurs for CO2 in the center of the 15-micron band, might not be found at any wavelength for some cases, such as clouds and water vapor).
An increase in solar forcing heats both the stratosphere and troposphere. The initial stratospheric equilibration for solar forcing is opposite that of greenhouse gas forcing in general (although there is an interesting similarity in the meridional temperature gradient). By considering tropopause level forcing with equilibrated stratosphere, that difference is accounted for and so the response to such a given tropopause level forcing will be similar for different sources of forcing provided that the forcing is not too idiosyncratic (regional patterns, such as for tropospheric aerosols, etc.) – there could be some difference via solar effects on the ozone layer, … stratospheric ozone depletion and volcanic cooling have a few effects similar to greenhouse and maybe solar warming (variations in NAM and SAM indices), …
I Thank Patrick 027 for his post.
I had typed out a long reply, but due to BUtterFingEers, lost it into the Eternal Bit Bucket!
I think it is important to distinguish “heat” and “energy” and “energy flux” – it is easy to get confused.
Chris stated in his main article:
“It is really important (in fact, essential) to understand that the greenhouse effect requires colder air aloft to work with, as you essentially replace strong surface radiation with weaker emission from higher layers. This is why adding CO2 creates an energy imbalance at the top-of-atmosphere. In an isothermal atmosphere, you could not get a greenhouse effect.” and
“Because the atmosphere is now emitting from colder levels of the atmosphere, the OLR has decreased, and the result is that the planet must warm to re-establish radiative equilibrium.”
While not necessarily agreeing with that statement, I will stipulate that if the CO2 horizon is in the Troposphere, then an energy imbalance will occur at the radiative top of the atmosphere.
But if it lies above that, if it is in the tropopause or stratosphere (and my numbers indicate that the lowest part is probably in the tropopause), then you don’t have “colder air aloft” – you have hotter air aloft. So the planet doesn’t have to warm up, it has to …cool down! (actually I think that the change would be very slight and there would be no great change in the average emission temperature).
Patrick 027 seems to imply that the CO2 emission horizon is indeed above the troposphere. Is that true, or am I missing something? (highly likely, but I can’t quite put my finger on it…)
I received this response from Chris on another blog:
Thank you for the reference to my website at https://chriscolose.wordpress.com/2010/02/18/greenhouse-effect-revisited/. I shall try to clarify some issues which Colin has brought up here and in the comments there. If I do not check back here, I am happy to take comments/criticism/questions at my site.
First of all, distinguishing between the surface energy balance and the top of the atmosphere energy balance is indeed crucial. It may be somewhat surprising that the latter generally rules the roost when it comes to climate change, but there’s solid physical reasons for this to be the case, and the mathematical treatments of how this all works out can be found in upper-level textbooks on climate and radiative processes. The details of the coupling between the troposphere and the surface are not “magical processes” nor are they based on hand-waving as Colin states in 109. The troposphere is well-stirred by convection to stay near the appropriate adiabat. In equilibrium, both the TOA and surface budget boundary conditions must be satisfied, and actually, one generally does not need to know the details of the surface budget to compute the influence of added CO2 in inferring temperature change, since the coupling is so strong between the two. This is particularly true over much of the globe, except perhaps in regions where the evaporative term suddenly becomes existent or non-existent. For example, if the Sahara surface were made moister, the surface would cool even with a bit of added CO2. If a rather moist region suddenly became dry, this would amplify the surface temperature increase and so the details of the surface budget become a bit more important.
In assessing the fate of radiative fluxes from the surface to space., one must keep in mind that outgoing radiation is emanating not just from the surface, but from all layers of the atmosphere, since air has a temperature. Furthermore, where the “radiating height” is in the atmosphere is very wavelength dependent. If it is a region in the atmospheric window, say, from 8-12 microns, this region is essentially the surface. But in a strongly absorbing band, a viewer looking from space does not need to look very far down. I have put up two images which show this:
The first figure in the top link measures upwelling spectral flux at the TOA, while the second measures brightness temperature (just an inverted form of the Planck function). The second figure in both links is transmittance, which is essentially zero in the relevant CO2 bands. It can be seen easily in the top figure of the first link, that this CO2 band is dead smack near the peak of the Planck function for terrestrial temperatures and is therefore of strong importance in the energy balance of the planet. Colin asked in points 3 and 4 in post 110 about the “temperature” of CO2 emission which is shown better in the second link. The stratosphere is only a tiny blip in the curve, and as more and more CO2 is added, the stratosphere moves up and cools, so this is not something that starts to weaken (or “reverse”) the greenhouse effect. And a large point of my post was that as you continue to add CO2, the hole that CO2 makes in the outgoing spectra does not really deepen to colder values, but instead widens to lessen the atmospheric window region. For these regions, adding CO2 will continue to generate more and more warming.
Proper fractional comparisons of the greenhouse effect show that the contribution from water vapor to CO2 is more like 2/3 to 1/3 instead of the GHG effect being completely swamped by water vapor as several secondary internet sources always seem to assume. The 15 micron band (and a few microns on either side) is important here, but also because the upper layers of the atmosphere are considerably cold and dry.
Hope this helps
c
To which I responded:
I thank Chris for his post. I hope he will also be posting it on his site.
There many things I don’t understand.
1. All are agreed that the GHGs, CO2 and H2O in particular (I will ignore the others just for the moment) absorb most of the Surface Radiation. Where does this occur?
Using the transmission figures (cited in Post #110) it is clear that the majority of 15um energy absorbed by CO2 will be soaked up in the first 500m of the atmosphere. In fact the tables also imply that around half is soaked up in the first 30 metres. I don’t have the figures for water vapour, but it is unlikely to be different, judging by the transmission diagrams. So I think it is fairly safe to say that apart from photons in the far wings (there are not many of these) the Surface Greenhouse effect is complete within the first 500m.
2. The energy radiated by the surface is 390W/m^2. The amount of this escaping to space is 40W/m^2. The back-radiation to the ground from the atmosphere is 324W/m^2. So the radiant energy absorbed by the atmosphere is 26W/m^2. (=390-40-324). (Figures are from IPCC AR4, WG1. I would think most of these are in dispute in detail, but the general argument holds that very little radiant energy is absorbed by the atmosphere.) Absorbed radiant energy is immediately converted to sensible heat. Sometimes (infrequently) a GHG molecule is sufficiently excited (by collisions) and lucky enough to emit a photon before it is stripped of the photon energy by another collision. If the downward photons are not re-absorbed they form part of the back-radiation. Basically the gaseous stew in the atmosphere radiates energy in all directions. Close to the surface only the downward radiation is not all reabsorbed.
3. Chris and I are in dispute about the mode of energy transfer within the atmosphere – this is primarily convection in my view. The case of the plastic Greenhouse seems to support this assertion. Both Miskolczi and Nicol believe that, because the atmosphere is in Local Thermodynamic Equilibrium, there is zero net radiative transfer in any direction, except in the two boundary cases (the bottom and the top). We can probably leave the heat transfer mechanism to one side for the time being (as it is somewhat immaterial, and progress to the top of the atmosphere.
4. The IR radiators within the atmosphere are, by and large, CO2 and H2O – these two gases have to do all the work in getting rid of the atmospheric energy to space. The other gases are not equipped with the right energy modes, which is why they don’t participate in absorption or radiation. CO2 and H2O can only radiate in the same bands as they absorb. Consider a particular photon emitted by a molecule of water in the upwards direction. In order for it to escape to space it must not be re-absorbed by any water molecules. So as we go higher and higher in the atmosphere, the chances of the photon escaping to space are improved. There will be some point in the atmosphere, below which virtually all the radiation is re-absorbed and converted to sensible heat, and none emerges to space. Essentially there is a water vapour emission horizon for that frequency.
5. Where is that horizon? In the water vapour case there are very many variables (surface humidity and frequency are the main ones), but we can guess that for the strongly emitted frequencies, the majority of the energy being emitted to space comes from a band between 3000m and 5000m. It won’t be much higher than that, because most of the water vapour has condensed into clouds – there’s not much water vapour in the atmosphere above the clouds. The temperature is going to be in the vicinity of -10DegC.
6. For CO2 the situation is much more predictable. We know that for the strongest emission frequency band, it only takes 500m for at 1 atmosphere to absorb all the photons. Moving to the top of the atmosphere, the CO2 horizon for that frequency (ie the LOWEST point from which emissions can get out to space) is somewhere in the region of 17,000m. This is generally in the tropopause. Furthermore, we know the majority of emissions will be coming from a place which has only 5% of the CO2 Horizon thickness (as 25m is about where 50% of photons are absorbed at ground level), and I make that to be 45,000m, ie well into the Stratosphere.
7. To summarise:
A.. The only way atmospheric thermal energy can leave the planet is by emission of IR photons by one of the Greenhouse gases.
B. This occurs from different levels for each frequency.
C. In general, the vast majority will be water vapor transmissions (hotter gas, many more excitation frequencies), and the emissions will be from low down.
D. For the strong emissions from CO2, these are necessarily from very high in the atmosphere. For the 15um band, we are talking the middle of the tropopause and above.
8. I wonder if Chris can find the flaw in that train of logic.
[The table cited in paragraph 1 is, for wavenumber 650:
CO2 Concentration, atm cm: 0.2 0.5 1 5 10 100 1000
Transmission %: 75 61 48 16 8 0.1 0
I calculate 1atm cm of CO2 to be the equivalent of 25m of atmosphere at a concentration of 400ppm.]
There is generally a net flux of radiation at any location in the atmosphere because there is enough transparency for photons to reach across significant temperature variations between emission and absorption (or escape to space). This will not be true at every wavelength and within sufficiently thick clouds for any wavelength, but it is more generally true. LTE is a good approximation for the vast majority of the mass of the atmosphere excluding the photons; the photons are mainly in LTE with there source regions but often not in LTE where they are absorbed or at points along the way from emission to absorption.
In case it wasn’t clear, the importance of CO2 as a greenhouse gas is not so much the amount of radiation emitted to space (or across the tropopause level, net), but the amount by which the presence of CO2 changes those numbers.
To a first approximation, the optical thickness of an amount of CO2 decreases exponentially away from 15 microns, which means that once the central part of the band is saturated (it is at the tropopause level), a given doubling widens the width of the band that exceeds a given set level of opacity less than the saturated; approximately the same intervals have intermediate opacity, but the width of large opacity increases at the expense of the portions of the spectrum where radiation escapes to space from the surface or clouds and water vapor or other spectrally-overlapping gases. Air-to-air net radiant transfer is low when the air is transparent and also when it is too opaque for photons to reach across significant temperature variations; thus air-to-air net radiant transfer would not change much by increasing CO2 once the central part of the spectrum is saturated if the air were otherwise transparent, but net radiative exchanges between the surface and air, air and space, and surface and space, would be affected; with clouds and humidity, CO2 affects net air-to-air radiant transfer when clouds or humidity, etc, are involved either in emission, absorption, or both (CO2 can block radiation from water vapor or clouds from escaping to space or the stratosphere, etc.)
At a given wavelength with sufficient opacity, the flux from the surface will drop to zero over a short distance, but the total upward flux decreases less rapidly because of emissions from the air. But it generally does decrease because the temperatures of the dominant emission sources decline (global time average).
Thanks for this response, Patrick 027.
If I can paraphrase, I think you are agreeing with Chris and me that an increase in the concentration of CO2 will increase the height of the CO2 emission-to-space horizon.
You have introduced another factor, which is that the wing frequencies are also affected -the horizon of the weak radiation in these areas is also increased in height.
But I don’t agree that the 15um horizon is within the troposphere, based on the tables for transmission in CO2. That may be true for the far wings, but not for the majority of the emission band.
Hope I haven’t missed the point of your post – if so, let me know.
Okay, but just to clarify, nobody said the 15 micron horizon was within the troposphere. Increasing CO2 decreases the net upward LW flux at the tropopause level more than above the stratosphere, and this causes stratospheric cooling (which feeds back on the net LW flux at the tropopause level by reducing the downward LW radiation from where it was after the CO2 increase but before the stratospheric cooling); the effects near 15 microns contribute to this cooling by increasing net upward LW radiation above the stratosphere while having essentially no effect at the tropopause level. The reason it has essentially no effect is that the horizons for radiation reaching the tropopause from either side are close enough (relative to the vertical scale of temperature variation) to the tropopause that further shifts toward the tropopause have little to no effect at such wavelengths with such great opacity.
The diagrams Chris cited above at https://chriscolose.files.wordpress.com/2008/02/upweeling_brightness.png are very interesting.
The temperature of the emissions in the main CO2 band is between 220 and 230 DegK, ie between about -47 and -57DegC. This places the median point of the emissions somewhere either:
A. In the high Troposphere to Tropopause, or
B. In the Tropopause to low Stratosphere
This post is in response to Patrick 027 at 1147, 04 March.
When you have a look at the details of radiation from gases it immediately becomes apparent that radiation is a very chancy affair. For most molecules excited to the emission energy state by collision with other molecules, there is insufficient time to release the photon before another collision occurs – only about one chance in 10,000 of emission, and 9,999 chances of not emitting.
The emissions arise because the gas is hot. IE the thermal energy within the gas is the cause of emissions which are enabled by collision.
It is not of much consequence how the gas got to be the temperature it did – it could be by NET absorption being greater than emission, it could be by convection, it could be by magic.
If CO2 doubles the 15um emission horizon moves higher – probably still within the tropopause. But the bulk of emissions will be further into the Stratosphere, so the intensity of emission will increase above the Tropopause.
I think what Patrick is saying is that this will cool the lower Stratosphere, effectively increasing the height of the top of the Tropopause. Yes, but I don’t buy that heating the atmosphere.
He claims that no-one stated that the 15um horizon was within the Troposphere. What then are we to make of Chris’s explicit statement:
“Because the atmosphere is now emitting from colder levels of the atmosphere, the OLR has decreased, and the result is that the planet must warm to re-establish radiative equilibrium.”
I’ve looked at the absorption numbers, and even in the far wings at wavenumbers 600 and 750, the bulk of the emissions (more than 50%) are above the Tropopause. So for virtually the entire CO2 emission band the atmosphere will be emitting from a warmer or just as warm place.
As Chris so eloquently put it:
“In an isothermal atmosphere, you could not get a greenhouse effect.”
The bulk of the CO2 emissions are coming from the isothermal portion of the atmosphere.
1. The shift in tropopause location has an effect but that is not the main cause of warming.
2. Even if more than 50 % of emissions to space are from above the tropopause, that can still leave a nonzero fraction originating from below.
Eyeballing the graph for 390 ppm CO2 above, the CO2 band has a significant effect over a width of ~ 200 /cm, while the width of the portion in the center where increasing opacity either increases emission to space or has little effect is about ~ 50/cm, leaving about 150/cm (adding both sides) where increasing CO2 will decrease outgoing LW radiation to space. There would be increased LW emission to space from the stratosphere or parts thereof over the whole CO2 band, which combines with a decrease in LW radiation from below the tropopause and increased absorption of that radiation within the stratosphere to produce the total effect on outgoing LW radiation. Far enough from the center of the CO2 band, upward emission from the troposphere increases, but there is a greater increase in tropospheric absorption of radiation from the surface.
As CO2 increases, the band spreads out; the width of the central portion increases, the width of the spectrum outside the CO2 band decreases; the width of the portion with intermediate opacity doesn’t change much for a range of CO2 concentrations, and continues to be the location at which changes in CO2 has the greatest effect on tropopause level forcing.
Near the CO2 band, water vapor contributes almost no opacity to the stratosphere.
3.
Actually, an isothermal atmosphere can produce a greenhouse effect if it is colder than the surface temperature, but that would be a short-lived condition, since this implies some convective instability near the surface. What Chris presumably was refering to was an atmosphere that is isothermal and isothermal with the surface.
But for the heck of it, consider an isothermal atmosphere colder than the surface. There can be a greenhouse effect by blocking surface radiation to space, replacing it with a smaller amount of atmospheric radiation; the effect saturates when the atmosphere blocks all surface radiation.
Under some un-Earthly conditions, scattering could become a more important contributor to the greenhouse effect. It works somewhat the same, by increasing opacity, but there is an important difference. For a greenhouse effect based only on scattering, the temperature of the atmosphere would have no direct effect on the radiation budgets.
Correction! In my last post I meant -43 to -53DegC.
Chris, thanks for the great post.
I found the following titles for Grant Petty:
1) “A First Course in Atmospheric Radiation.”
2) “A First Course in Atmospheric Thermodynamics.”
Response: I have both, and they are very good reads (I was referring to the first in my previous comment). As it happens, Petty is a faculty member at UW-Madison where I am receiving my Atmospheric & Oceanic science education, so naturally people like to keep books within the department if possible. The thermodynamics book is meant to somewhat precede the radiation book, but you can still read the latter on its own. He does a good job of making the material qualitatively understandable and intuitive, lots of examples, analogies, and applications, while still keeping enough mathematics to be appropriate for an upper-level undergraduate science major or beginning grad student in the field– chris
Pingback: Almost everything you wanted to know about the greenhouse effect « A Man With A Ph.D.
While G&T is off topic, actual physics is not; I hope Chris doesn’t mind if I post a quick wrap up of something from another thread (I wouldn’t bother, but when I make a comment, and then see some parts that deserve clarification, I want to make those clarifications)
end of second to last paragraph of
Which means that the net flux (I forward minus I reverse) between any two pairs of locations, from the contributions to emission and absorption, is always from higher to lower temperature.
(bold added after original posting)
‘flux’ was not the word to use there; I was refering to the net intensity; and since I was refering to an intensity between emission and absorption, it must be the intensity per unit volume, but for clearer comparison, we can multiply that be a small unit volume dV at each location (dV1 and dV2) and thus simply refer to the net intensity from one to the other: specifically, the net intensity per along ‘a’ path from one location to another; when scattering, reflection, etc, occur, this path might branch or diffuse into huge multitude of paths in between the points, but I am refering specifically to: 1. all the contributions to the net I coming at one location from one direction that originate from the other location through a direction at that location; 2. that can be summed over all directions at just one location to give the total net intensity in one direction at the other location that is to or from the first location; 3. that can be summed over all directions at the other location to give the net energy flux from one location to the other (from one unit volume to the other; in this case it is not a flux per unit area, but just a flux).
This should also be clear from the last paragraph.
…
And:
I used ‘unit ___’ incorrectly a few times; a unit ___ is an amount = 1 [unit]; when I refered to dx and dV as unit length and unit volume, that’s incorrect. They are ‘small bits’ of length and volume, or more precisely, differential lengths and volumes (the limit as size goes to zero without actually being zero); of course, a ratio of d_ / d_ = __ per unit ___ if there is a relation.
Very good article–technical but accessible.
I did have one question, though–you mention several times that the temperature response to CO2 is logarithmic, but the graph you have for that function does not appear to conform to a limit on the y-axis at the bottom of the graph. Why is that? Math was never my strongest suit, but I always thought that a logarithmic function meant that increases in A would always give vastly diminishing changes in B, to the point that the change in B always approaches zero.
Thank you.
Response: The logarithmic relation has to do with the manner in which the absorption coefficient decays (exponentially) away from the center (see the Angstrom article). It does give you a diminishing effect– which is why going from 300 ppm to 600 ppm has the same effect as going from 600 to 1200 ppm (instead of going from 600 to 900 ppm)– but it never truly goes to zero. In reality, if you were to put sufficient amounts of CO2 in the atmosphere (say 10 to 20% of the current atmosphere), the response actually becomes larger than at current concentrations because weaker absorption bands that have different structures than those which dominate the present climate become important, and you also start to get pressure broadening effects which makes CO2 a more efficient greenhouse gas. That’s obviously not really important for modern global warming, but even one or two doublings right now is something to worry about as that can be severely disruptive to socio-economic and ecological structure– chris
Very nice article, thanks.
Fantastic post, Chris! This deserves to become a classic among clear expositions of some of the basic science of climate change. I know that I will refer back to it often!
Chris says:
“It is really important (in fact, essential) to understand that the greenhouse effect requires colder air aloft to work with, as you essentially replace strong surface radiation with weaker emission from higher layers. This is why adding CO2 creates an energy imbalance at the top-of-atmosphere. In an isothermal atmosphere, you could not get a greenhouse effect.”
In the 15um band, which is the most relevant and strongest CO2 emission/absorption band, all of the emissions to space are coming from the Stratosphere. This has a POSITIVE temperature gradient with height, not a negative one, so the Stratosphere would tend to COOL.
Previous commentators have claimed that the radiative transfer across the Tropopause into the Stratosphere means that the lower atmosphere has to heat up. But the Tropopause contains a large proportion of the atmosphere – around 20-25% by my reckoning. This is optically thick at most of the GHG frequency bands, so very very little radiation can get across the Tropopause to the Stratosphere.
Essentially the Stratosphere is not warmed from below, but from above.
So I reckon that addition of CO2 will COOL the Stratosphere, and has no effect on the lower atmosphere.
Pingback: Greenhouse effect revisited. - The Environment Site Forums
Chris, these observations from Roger Taguchi are relevant to the topic of this thread. They were recently posted on Eli Rabett’s “Eli can retire ..” thread at http://rabett.blogspot.com/2010/04/eli-can-retire-part-viii-epa-reads.html .
QUOTE:
Re the balance between incoming short-wavelength incoming solar radiation (visible light) and outgoing long-wavelength IR (infrared), it seems to me that all the mathematical equations in the literature ASSUME radiative equilibrium. The difference is then the greenhouse effect. No one except Nicol (and me) seems aware that there are non-radiative mechanisms (i.e. collision between molecules) for energy transfer between molecules. Thus all the equations for radiative exchange miss the fundamental mechanism for the greenhouse effect: greenhouse gas molecules (CO2, H2O, methane, etc.) absorb some of the IR emitted by the solid and liquid surface of the Earth by jumping to an upper vibrational energy level (with vibrational quantum number v = 1) and transfer this excess vibrational energy (at equilibrium at 200-300 K, the molecules would almost all be in their ground vibrational states, with vibrational quantum number v = 0) BY COLLISION to air molecules (N2, O2, Ar) which CANNOT re-emit IR radiation (because they have zero changing electric dipole moments).
Thus BY COLLISION the energy gets exchanged with other air molecules, and the atmosphere gets hotter than in the absence of greenhouse gases. This fundamental mechanism has been obvious to me for over 4 decades (when I was a high school student around 1960, the encyclopedia “explanation” for the greenhouse effect did not totally make sense to me, since I could not see how changing visible light energy to IR would warm the atmosphere; studies in Physical Chemistry then allowed me to independently understand the greenhouse effect, and then further grad work on energy transfer during collision by excited molecules in Nobel laureate John Polanyi’s group reinforced my understanding). Has increased specialization in academic education reduced general understanding of fundamental physics so much that no one else can see the nonsense in the “220 K blackbody radiation from the upper troposphere”, supposedly the “explanation” for the greenhouse effect? UNQUOTE.
EliRabett responded with QUOTE: Roger and you should go read Goody and Yung. What you write is well known and known in detail. Oh yeah, we can exclude Richard Courtney and Jack Barrett. UNQUOTE.
Here is a relevant response which Roger Taguchi made on Australian Senator Fielding’s “Is global warming man-made? Is global warming dangerous?” thread (Note 1). This should help to shed more light on this poorly understood topic QUOTE:
.. thank you for showing me a good explanation involving the importance of energy transfer on collision between excited CO2 molecules and surrounding air (N2, O2, Ar) molecules, in agreement with my own independently-arrived-at view. This should be emphasized in textbooks on climatology (which I admit to not having read, most of my information to date coming second- or third-hand from wikipedia and this website).
.. it seems to me that the accepted explanation of the greenhouse effect then goes on to say that IR emitted from the solid and liquid surface of the Earth is exchanged using CO2 molecules at 300 K or so (at the surface) to an ultimately-emitting layer in the upper troposphere at 220 K. This is where we differ about the mechanism. For instance, if the Earth had absolutely no CO2, including in the upper troposphere, would there be a 220 K blackbody-emitting layer? I say no, because N2, O2 and Ar do not have changing electric dipole moments, and therefore cannot emit IR radiation. But by the accepted explanation, with no CO2, there would be even less outgoing radiation, and therefore an even higher greenhouse effect (see any college textbook on physical chemistry for the derivation of Planck’s blackbody radiation formula, which would be applicable for an opaque = black surface, not a transparent atmosphere), a logical contradiction.
The accepted explanation is also disproven on inspection of the satellite spectrum looking down on Antarctica: the surface of Antarctica is at or below 200 K, and yet there is an observed emission at CO2 frequencies GREATER THAN the total blackbody surface emission, and this assumes even at zero net absorption by CO2! This is contrary to the Second Law of Thermodynamics (NET heat flow does not occur from a cold to a hot surface). Lamely explaining the emission over Antarctica as due to a “temperature inversion” without explaining how the molecules at altitude gained that energy is no explanation (or equivalent to saying that summer temperatures are higher than winter temperatures because the thermometer readings are higher in summer).
I have explained the origin of the CO2 emission measured by satellite over Antarctica in a longer article I can email on request to rtaguchi@sympatico.ca. The manuscript is in WordPerfect (Version X3), .. but in case your computer cannot decipher WordPerfect, I can email a scanned pdf file. Briefly, the CO2 emission seen over Antarctica (and even more so over the rest of the Earth) is caused by IR frequencies in incoming solar radiation which boost ground state molecules to much higher vibrational levels (e.g. to v=3 in bond-bending mode). These excited molecules can then emit longer wavelength IR photons, in particular the observed CO2 radiation at 670 wavenumbers, as they cascade down to the ground state one vibrational level at a time (e.g. from v =3 to v=2, then from v=2 to v=1, and then from v=1 to v=0). The incoming blackbody solar radiation in the upper atmosphere has enough energy at the relevant frequencies to explain the observed outgoing CO2 emission.
UNQUOTE.
NOTES:
1) see http://www.stevefielding.com.au/forums/viewthread/795/P855/
Best regards, Pete Ridley
Absolutely none of that brings to light anything that is not already part of climate/atmospheric science.
In the vast majority of the atmosphere (by mass and thus, with some adjustment, by optical thickness), the process of thermalization (via molecular collisions) is rapid enough relative to photon emission and absorption, that when a population of molecules emits or absorbs radiant energy, the change in energy is shared by all the molecules in a given volume (with enough particles for statistical significance, yet small enough to be nearly isothermal), so that each non-photon constituent (CO2, H2O, N2, O2, Ar, CH4, etc.) is at nearly the same temperature, with the distribution of energy characteristic of local thermodynamic equilibrium – so that the matter can be described as having a single temperature, and will emit photons accordingly for it’s optical properties.
Thus, when photons are absorbed, the temperature (and enthalpy) of a volume of air increases; when photons are emitted, the temperature (and enthalpy) of a volume of air decreases, regardless of which molecules are responsible for emitting and absorbing the photons. If emission and absorption occur at the same time, their is a net energy loss or gain. If the volume of air is emitting as much energy as it is absorbing, then absent other processes (temperature changes via adiabatic expansion or compression, or latent heating or cooling, or, if within ~ 1 mm of the surface, conduction of heat to/from the surface), the temperature stays constant.
Any given layer of air, and the surface, may gain heat from solar radiation, may also absorb energy from LW emission from the surface or another layer of air, and may also emit LW radiant energy to the surface or to other layers of air or to space. The optical properties affect the density of emission and absorption and the distances over which photons reach between emission and absorption.
The climate system as a whole tends to gain or lose heat energy if solar heating and LW cooling to space are not balanced. In particular, a layer that is recieving more heat than it is losing will tend to increase temperature, which will tend to increase LW emission, which will increase the heat loss; etc. for the opposite case.
The tropopause is a boundary between the troposphere and the stratosphere; one could consider a particular layer to be the region of the tropopause, but the troposphere and stratosphere are actually in contact with each other at the tropopause. Radiant fluxes through the tropopause include those from above that are absorbed below and those from below that are either absorbed above or go to space.
—–
Solar heating is not uniform over the globe. But any part of the surface or atmosphere not at zero K will emit LW radiation. The air above polar regions, especially in winter, is warmer than otherwise because of horizontal convection from lower latitudes. That is a source of energy for greenhouse gases that emit LW energy to space.
See also (link pending)
I agree pretty much with Patrick 027’s contribution.
The only point I would like to stress is that the Tropopause thermally and radiatively (as far as the CO2 frequencies are concerned) isolates the Troposphere and Stratosphere.
There can be no net energy transfer from the Troposphere to the Stratosphere by convection (needs a temperature gradient) or by conduction (needs a hotter conductor than conductee).
The Tropopause is deep, and contains around one fifth to one quarter of the atmosphere. Neglecting the low energy wings of the bands, most photons emitted by CO2 above or below the Tropopause won’t make it through. The net radiative energy transfers will be from the Stratosphere into the tropopause and the Troposphere into the Tropopause (cos it’s the colder body).
I don’t agree that CO2 emissions are getting to space from the Troposphere, except in the far wings of the bands. The absorption by CO2 in the main (most energetic) 15um band is very fierce, so any emission from as low in the atmosphere as the Troposphere will be extinguished well before it gets to space by the overlying CO2 molecules. Only when emissions come from the Stratosphere will there be any significant emission to space, as there is so little overlying absorbant GHG.
Colin, the tropopause is usually defined as a boundary, not given significant thickness in it’s own right. (Is it possible you are thinking of the lower stratosphere, which at latitudes outside the tropics tends to almost isothermal with height?)
Within the atmosphere, conduction (and diffusion of water vapor) are very ineffective, relative to convection and radiation, at transporting heat over large distances; they are really only important within about 1 mm of the surface and maybe in the small-scale vicinity of growing or evaporating or freezing or melting cloud droplets/crystals.
Radiation does transport heat up through the stratosphere from below and some of that is absorbed. Much of the radiative exchange between the stratosphere and troposphere may be accomplished in wavelengths dominated by water vapor. The effect of CO2 …
… continued below
“Solar heating is not uniform over the globe.”
Consider a 1-dimensional model with solar radiation averaged over the day and year.
For a strictly emitting/absorbing greenhouse effect (LW scattering can also contribute to a greenhouse effect but the physics is a bit different; in particular, atmospheric temperature would be inconsequential in a purely scattering greenhouse effect, wherein all emission to space originates at the surface; the scattering effectively reflects a portion of the upward flux back down; however, with a mixture of scattering and absorption/emission, greater scattering can have some of the same effect as greater emission or absorption, by concentrating the weighting functions of emission and absorption):
Start with no LW emission from the atmosphere. In this case, all the LW cooling to space comes from the surface, and so the surface temperature tends towards an equilibrium as determined by solar heating, regardless of how solar heating is distributed (all the solar heat deposited in the atmosphere has to be transfered to the surface before it can be emitted to space.
In this situation, solar heating of the atmosphere will make the atmosphere warmer than the surface.
—-
Let’s establish 3 different levels at which to measure the forcing: TOA (top of atmosphere), TRP (tropopause) and SFC (surface), and these can be instantaneous (i) or after stratospheric equilibration (seq). The forcing is a change in net downward radiative flux; a reduction in upward flux or increase in downward flux adds to forcing.
When there is no troposphere, the tropopause level can be considered to be at the surface (and surface+troposphere is the surface), so TRP forcing = SFC forcing. This is the case for zero greenhouse effect as described above.
Now consider the forcing of adding a little bit of LW optical thickness. IF the atmosphere is initially isothermal with the surface, then TOAi forcing = 0; if some of the solar heating occurs in the atmosphere, then the atmosphere may be warmer than the surface, so that TOAi forcing < 0. If the surface is not a perfect blackbody, then TOAi forcing will be smaller than otherwise.
But the TRPi and SFCi forcings, which are equal, will be positive, because of an increase in the downward LW flux from the atmosphere.
The difference in forcing between two levels is equal to a net radiative heating or cooling of the space in between. Positive forcing below and negative or zero forcing above implies radiative cooling of the layer in between.
—-
The atmosphere cools. This 'stratospheric equilibration' reduces the LW emission to space and the downward LW flux to the surface+troposphere; this increases TOA forcing and decreases TRP forcing. After this, TOAseq forcing = TRPseq forcing. But TRPseq forcing, though smaller than TRPi forcing, is still positive, because there is still some downward LW radiation from above TRP, whereas there was none before.
Temperature changes that occur higher in the stratosphere will affect the TOAseq forcing more than the TRPseq forcing, and vice versa for temperature changes that occur lower in the stratosphere; however, if, of the wavelengths (weighted by the Planck function) where LW optical thickness is not too small, it is not much, then the stratospheric equilibration will change TRP and TOA forcing by about the same magnitude, because the source of emission in either direction is distributed over the whole layer.
To achieve full climate equilibrium, setting optical property feedbacks (water vapor, clouds, snow and ice, vegetation, etc.) aside, the temperature of the surface+troposphere must increase to produce an increase in upward LW flux at TRP to balance the TRPseq forcing plus the additional downward LW flux that occurs in response to the stratospheric temperature increase that results from the increased upward LW flux from below plus any solar heating that is sequestered into the troposphere as a result of tropopause level height increases, minus any decrease in downward LW flux due to the same (PS so far as I know, these sorts of feedbacks are generally minor compared to water vapor, clouds, snow and ice, vegetation, etc. – ?).
—-
When the temperature distribution required for radiative equilibrium (wherein each level emits as much as it absorbs) results in a temperature decrease away from the surface that is greater than a convectively-stable lapse rate, convection tends to occur, transporting some heat vertically from the surface to parts of the atmosphere, and keep the temperature change with height over this region near that of an adiabatic lapse rate. The dry adiabatic lapse rate (change in temperature with change in pressure with no net radiative or latent heating or cooling or mixing or conduction) does depend on temperature (and pressure), but of greater significance, the moist adiabatic lapse rate, which is the rate of temperature change with height that occurs if latent heat is released as condensation removes water vapor to keep relative humidity at 100 %, depends on temperature; thus, as the temperatures of the surface+troposphere change in response to any radiative disequilibrium (forced or internal variability), their is a lapse rate feedback in the relationship of surface temperature to the overall change. (Concievably, there could be other ways to alter the lapse rate, because in an actual single atmospheric column that is in radiative-convective equilibrium, the lapse rate would be dry adiabatic in between the surface and the cloud base; a change in relative humidity at the surface would affect this).
But the changes in temperature at any level within the troposphere will tend to be related to changes in other levels by the convectively-maintained lapse rate. This is because, if the radiative heating and cooling changes so as to heat some level or cool another level, the resulting temperature changes destabilize the atmosphere above or below and stabilize the atmosphere, or otherwise reduce convective heat fluxes,the atmosphere below or above, respectively. The resulting changes in convective heat flux spread the temperature changes over the troposphere, to or from the surface. LW fluxes can do the same to some extent (vertically spread out the effect of a localized forcing or feedback), as implied by the effect of stratospheric equilibration on TRPseq forcing and the effect on the stratosphere of tropospheric and surface warming, but the convection in the troposphere tends to maintain a particular lapse rate.
Thus, the troposphere+surface response to radiative forcing tends to follow TRPseq forcing (+ radiative feedbacks in net flux the TRP level) similarly at all vertical levels according to the physics of convection. This isn't to say that the distribution of radiative heating or cooling below TRP doesn't matter to climate; changes in the distribution of radiative heating or cooling within the surface+troposphere can have effects, such as in requiring changes in convection (which could affect radiative feedback at TRP).
——–
What about the full 4-dimensional climate? – there are forced annual and diurnal cycles in solar heating, as well as latitudinal and regional/local variations in solar heating. There are also fluctuations over short time periods within the longer-term climatic state.
1. Conservation of energy still holds true, and the vertical levels, including the TRP (interpolating through breaks such as at jets), form closed surfaces around the globe. Thus in the global average, there are no horizontal fluxes to remove or add heat. Averaged over time, for a steady climatic state, the storage terms have to be zero, so the net vertical fluxes still have to balance. In this respect, a 1-dimensional model still describes key aspects of the system.
However, the relationships of radiative fluxes to global averages in temperature and optical properties are more complex – variations and their correlations have an effect, though this might (?), at least in so far as temperature is concerned, only involve small corrections to the calculation of fluxes based on global average conditions (?) (for example, because of the nonlinear relationship of LW emission to temperature, variation in temperature tends to result in greater emission for the same average temperature. But the effect is small; a crude estimate on my part suggests combined regional and temporal temperature variations at the surface would make the surface, assuming constant LW emissivitiy (not actually true) with an actual global average of 288 K radiate the same total LW flux as a 289 K isothermal global surface – a 1 K difference, which doesn't completely appear or dissappear for climate changes of several K at least).
2. Also, the way convection maintains the lapse rate is more complex.
Their are regional/latitudinal/diurnal/seasonal forced variations in solar heating, and the climate system responds to this with variations in temperature and circulation.
Though not necessarily always distinct and not independent of each other, convection can be considered to occur in two forms – large horizontal scale overturning (LHSO) and localized vertical overturning (LVO). Convective motion can be thermally direct (warmer air rising), converting some heat to kinetic energy, or thermally indirect (warmer air sinking), which must be forced by kinetic energy and converts kinetic energy to heat.
Kinetic energy can also be converted to heat in the process of mixing air against stable stratification, which is analogous to and involves thermally indirect motion, and it can be converted to heat by viscosity/friction. Thus kinetic energy in some ways is like latent heat, accomplishing part of the convective heat flux, though it is a relatively small part. Some kinetic energy produced in the troposphere+surface propagates into the upper atmosphere, and there is some slow overturning (driven by that kinetic energy) of air within the upper atmosphere, with some transfer of mass to and from the troposphere; both of these allow some convective heat transport to or from and within the stratosphere and above, but it is, at least in global-time average effect, relatively small, so a pure radiative equilibrium in the global seasonal average can still approximate upper atmospheric condititions (though there are significant regional/latitudinal deviations from radiative equilibrium).
LVO can occur spontaneously when and when the lapse rate is unstable to moist convection; however, moist convection doesn't occur automatically in such conditions; it can require triggers. (It can also be forced by the wind-driven mixing, in particularly near the surface, which actually transports heat downward.)
LSHO can occur spontaneously even if the atmopshere is stable to all LVO. Thermally-direct LSHO takes such forms as the Hadley cells, Walker circulation, monsoons, and extratropical synoptic-scale eddies/waves (storm track activity) (** but some LVO type convection is often associated with areas of larger-scale ascent in LSHO circulation). Thermally-direct LSHO transports heat horizontally from higher to lower temperatures, and also transports heat vertically, so it can maintain a lapse rate that is stable to LVO. Reduced horizontal temperature gradients and higher local static stability can reduce the available potential energy to such motion, so as with LVO, the rate of LSHO is limited ultimately by differential diabatic heating/cooling, in this case vertically and horizontally. A change in horizontal variations could affect LVO by changing LSHO.
Relative to the density of net radiative heating/cooling, most of the atmosphere, as well as the ocean, has enough heat capacity to keep temperature changes small in response to diurnal variations in solar heating. The land surface can undergo a larger diurnal temperature cycle (effective heat capacity is limited by the rate at which heat can conduct through the surface material). Air and water can also travel into regions with different radiative conditions, and their temperature will respond, but over a time scale proportional to heat capacity. Thus, temperatures reflect prior histories of radiative and convective conditions. Sometimes, over time and space, cooler surface or air will form or move under warmer air, inhibiting LVO; Sometimes the opposite may occur, encouraging LVO. The air temperature over the globe, especially away from the surface, may tend to follow changes in temperature at the surface more in regions and times of higher temperature (and humidity) than in regions and times of colder temperature. Radiatively-driven temperature changes in regions with greater vertical stability can have more vertically-concentrated effects.
Still, the troposphere and surface as a whole tend to respond to globally-averaged TRPseq forcing and feedbacks, with a preffered pattern according the the variations in stability and feedbacks (such as ice-albedo) and moist-adiabatic lapse rate feedbacks, and perhaps (?) changes in vertical convection due to the radiative effects of water vapor (and clouds), if the forcing itself is not too idiosyncratic. LVO spreads the temperature changes vertically where it can; LW responses do the same elsewhere, and the large-scale circulation of the atmosphere (and also the ocean) spread changes horizontally. The whole system is connected.
In regions with temperature inversions at/near the surface, where the surface is colder than at least some portion of the air above (heated either by ealier conditions or at a different location), there may be, depending on clouds and water vapor, a net LW heating of the surface by the air, or at least that layer of air. At the same time, depending on clouds and water vapor, the surface may have a net LW cooling to other layers of air and/or space. There can also be a net convective heat transfer to the surface from the air immediately above, which may be recieving a net LW flux from warmer air higher up. The surface can get or stay colder than at least some of the air above because of radiative cooling to other air and space, but how cold it can get or stay is limited by the heat the surface gets from some layers of air, heated at an earlier time or a different place.
An increase in the greenhouse effect can reduce the surface cooling to space or colder air at higher levels, and warming at other locations or times can keep the surface warmer than otherwise by the increased heating from some of the air above. At such locations and times, the surface temperature will still tend to follow at least somewhat the overally surface+tropospheric trend, and could even have enhanced warming if an increase in radiative heating is concentrated near the surface, as the lack of LVO doesn't immediately spread the effect vertically (though horizontal motions and the passage of time will spread the effect at least somewhat in space and time).
Patrick 027 said “Colin, the tropopause is usually defined as a boundary, not given significant thickness in it’s own right. ”
I thank Patrick for his observation, and particularly his subsequent remarks that the Tropopause, as with all things in the fluid atmosphere, is a very variable beast.
I have been using the International Standard Atmosphere (see http://en.wikipedia.org/wiki/International_Standard_Atmosphere) as my model. This defines the Tropopause as a region from 11 to 20km at -54.5DegC.
Patrick also said: “Radiation does transport heat up through the stratosphere from below and some of that is absorbed. Much of the radiative exchange between the stratosphere and troposphere may be accomplished in wavelengths dominated by water vapor. The effect of CO2 …”
[I prefer to use the term “energy” rather than the much looser “heat” (which is actually defined as an energy flow or transfer) preferring to reserve the latter term for “sensible heat” or temperature.]
If the Tropopause is as thick as the International Standard Atmosphere says it is, then it has an appreciable amount, about 25-30% of the mass of the Atmosphere. There is hardly any water vapour, so any emissions at water vapour frequencies will not be greatly absorbed by the Tropopause. It is not until the temperatures start to increase above -40DegC in the high Stratosphere (around 30km) that there is any appreciable Water Vapour. There’s still not much – the air is very thin, but the water vapour content does increase with altitude, so we would expect that the higher Tropopause is indeed absorbing some of the photons emitted by Water Vapour in the Troposphere.
The Tropopause/ Lower Stratosphere is too thick in the International Standard Atmosphere to have many CO2 emitted photons make it through from the Troposphere to the Stratosphere in either direction.
“It is not until the temperatures start to increase above -40DegC in the high Stratosphere (around 30km) that there is any appreciable Water Vapour.”
Right idea, wrong application.
Water vapor concentration, as a mass or molecular (molar or volume) fraction of the air, decreases away from the surface and it highest near the surface in warm conditions if there is sufficient moisture supply upwind or onsite. Rising motion results in cooling, which, when relative humidity (vapor pressure / equilibrium vapor pressure over a flat surface of pure water) reaches ~ 100 %**, converts vapor to liquid or solid form (which releases latent heat, reducing the rate of cooling). This may again evaporate upon sinking or mixing with dry air (the later case being important in severe thunderstorms and tornadic activity), and some precipitation can remove condensed water to deposit it in lower-lying air (virga), but (so far as I know) much of the condensation in the atmosphere corresponds to precipitation reaching the surface nearby, so the outflow from moist updrafts, upon sinking back to higher pressures and temperatures, can be quite dry, as much of the condensed water has been removed and isn’t available to evaporate into the air.
There is some injection of water into the stratosphere by convection, and some supply by the oxydation of methane. It is a small amount but it is optically significant in some wavelength bands. Stratospheric water vapor isn’t generally removed by condensation within the stratosphere with precipitation from there; it simply gets back into the troposphere eventually in vapor form. Thus the changes in temperature within the stratosphere don’t have the strong direct effect on water vapor within the stratosphere that they do in the troposphere, and the relative concentration of water vapor doesn’t vary so much with height above the tropopause.
**-the actual process is more complex in detail and tends to involve some initial supersaturation; see Kohler curve, cloud droplet, haze particle
______________
“I have been using the International Standard Atmosphere”
Okay, but in textbooks the tropopause is simply a boundary between the troposphere and stratosphere. But it is not a fixed boundary, that is true. It is lowest at high latitudes in winter, where I think it actually gets below 10 km (maybe 8 km?). 11 km is a typical height in midlatitudes. In the tropics it can/does get ~ 16 km or higher.
Patrick 027 said:
” Thus the changes in temperature within the stratosphere don’t have the strong direct effect on water vapor within the stratosphere that they do in the troposphere, and the relative concentration of water vapor doesn’t vary so much with height above the tropopause.”
I thank Patrick for his detailed response.
I agree that water vapour is stripped out of the atmosphere as you go higher in the troposphere, because the air is colder. The (average) 78W/m^2 of latent heat (3 times the radiated transfer) goes back into the atmosphere as the vapour condenses, mostly I think in the clouds.
So The Stratosphere shouldn’t have any. But it does, and in higher quantities than the upper Troposphere. I had a look at some radiosonde data. It shows that there is an appreciable increase in water vapour with altitude in the Stratosphere. For example, WV content at 11km and -62.5DegC was 0.02g/kg. At 16km and -56.5DegC it was 0. At 20km and -61DegC it was 0. At 26km and -57DegC it was 0.01. At 30km and -54 it was 0.03. At 34km (end of record) and -44 it was 0.11. The water vapour is all gone by the Tropopause but re-emerges, increasing with height in the Stratosphere.
http://www-das.uwyo.edu/~geerts/cwx/notes/chap01/tropo.html
not every detail here is correct, for example, the coldest part of the atmosphere is not the tropopause where it is highest (which is in low latitudes) or the winter stratosphere over Antarctica, although it is true these are the coldest places below the mesosphere – the actual coldest location is around the summer polar mesopause, where it gets down to below 140 K, or about -133 deg C;
but this part at least seems about right:
This at least broadly agree with what I remember reading and seeing in textbooks
(Hartmann, p.4 the equatorial tropopause is about 17 km; tropical tropopause is coldest place in the lowest 20 km (except maybe Antarctic winter stratosphere – but not near the tropopause there, see Holton);
Holton, p.404 shows (in the zonal average) the tropical tropopause at about 100 mb height, getting colder than 200 K (-73 deg C) in some latitudes;
Wallace and Hobbs p.27 (older resource) depicts (in the Northern Hemisphere, zonal averae) the tropopause near the equator just above 100 mb (near 17 km to a bit under 18 km, depending on season) and below -75 deg C (~ 198 K) or -80 deg C (~ 193 K), depending on season, near the equator, and at the poles, a little above the 300 mb level and below -50 deg C (~ 223 K) in July and near 300 mb and below – 60 deg C (~ 213 K) in January.
Bluestein, pp. 182-183; implies that the tropopause is typically near 1.5 PVU
(in the extratropics, I assume; in the extratropics, a ‘dynamic(al) tropopause’ can be defined at a particular potential vorticity, such as 1.5 PVU or 2 PVU (skimmed this, looks good: http://ocw.mit.edu/NR/rdonlyres/Earth–Atmospheric–and-Planetary-Sciences/12-803Fall-2009/RelatedResources/MIT12_803F09_PV_maps.pdf ) )
)
Class notes: the tropopause is about 17 km near the equator and about 7 km at the poles
————–
My explanation about stratospheric water vapor could have been a little clearer:
The point is that, knowing there is not much condensation or precipitation occuring in the stratosphere, water vapor is not generally removed from the air upon cooling. Either there is insufficient nuclei for condensation to occur, or the relative humidity at warmer conditions and lower pressures is low enough that saturation is not attained when that air is cooled and/or compressed (saturation vapor pressure depends on temperature; mixing ratio is conserved in the absence of mixing or precipition and condensation, etc, and for the same mixing ratio, partial pressures decrease with a decrease in total pressure; thus, the saturation mixing ratio increases with height if temperature is constant, and increases even more if temperature increases with height; the cooling that could occur upon descent (or movement to different latitudes) would be radiational, not adiabatic).
It makes sense that this should be the case if all water vapor entering the stratosphere comes through the bottom of the stratosphere, where the saturation mixing ratio tends to be lowest.
But some water vapor could be intraduced at higher warmer levels by oxydation of methane; if this were a large enough source, then air from higher and warmer parts of the stratosphere and above could, upon radiational cooling following descent or horizontal movement, reach saturation and form clouds – if sufficient nuclei were present (of course, clouds would contribute their own radiative properties to the mix). So far as I know, this doesn’t seem to be the case, though, at least not typically, at least not under present conditions.
Class notes on stratospheric water:
rates of addition of water to stratosphere:
Hadley cell: 0.22 trillion kg/yr
Severe storms: 0.8 trillion kg/yr
Oxidation of CH4: 0.1 trillion kg/yr
(compare to global precipitation: approx. 510,000 trillion kg/yr)
concentration of water vapor: 3 ppm
residence time: 1.6 years
—-
The circulation of the upper atmosphere on average is an upward motion out of the troposphere in low latitudes, and upward motion in the mesosphere in the summer high latitudes, and sinking motion in winter high latitudes; it is driven by fluid mechanical waves propagating upward from the troposphere. The adiabatic cooling and warming associated with this motion keeps the summer polar mesopause colder than radiative equilibrium and the winter high latitudes of the mesosphere and stratosphere warmer than radiative equilibrium.
Holton, p.404:
In the tropics, the temperature increases with height from the vicinity of the tropopause to the stratopause; at higher latitudes, the lower stratosphere is nearly isothermal or even has some temperature decrease with height in winter polar regions.
… of course, it is hard to define the tropopause with such precision that you could place a piece of paper on it; and so one could define a tropopause zone or layer (there are frontal zones too, although in that case, the whole of the frontal zone is different from what is on either side, whereas a tropopause layer would just be a transition between troposphere and stratosphere) but what is important is that there is a distinction between the troposphere and stratosphere; one way to avoid giving the boundary it’s own mass would be to describe the mass in such a layer as being a mixture of troposphere and stratosphere, though I don’t know if that would be practical; the description of the atmosphere I was presenting assumes the tropopause has no actual thickness, but I think it allows that it’s exact position might not be pinned down to the nearest meter. Anyway, it’s not 5 or 10 or 20 km thick.
(I’m going to continue discussions about the nature of the tropopause (if necessary) and water vapor at the end of the thread)…
… as the lack of LVO doesn’t immediately spread the effect vertically (though horizontal motions and the passage of time will spread the effect at least somewhat in space and time).
PS that would account for the high latitude warming being concentrated near the surface, but the radiative source of that warming includes a surface albedo feedback that is also concentrated into higher latitudes.
Roger Taguchi, via Pete Ridley, said:
“Briefly, the CO2 emission seen over Antarctica (and even more so over the rest of the Earth) is caused by IR frequencies in incoming solar radiation which boost ground state molecules to much higher vibrational levels (e.g. to v=3 in bond-bending mode). These excited molecules can then emit longer wavelength IR photons, in particular the observed CO2 radiation at 670 wavenumbers, as they cascade down to the ground state one vibrational level at a time (e.g. from v =3 to v=2, then from v=2 to v=1, and then from v=1 to v=0). The incoming blackbody solar radiation in the upper atmosphere has enough energy at the relevant frequencies to explain the observed outgoing CO2 emission.”
I agree. Based on the absorption figures, the 15um band is definitely being emitted by CO2 in the Stratosphere, and the most likely sources of energy are absorption of sunlight by CO2 and Ozone in the Stratosphere, which is almost completely thermally and radiatively isolated from the Troposphere by the deep and very cold Tropopause.
“which is almost completely thermally and radiatively isolated from the Troposphere by the deep and very cold Tropopause.”
No.
1. Atmospheric optical thicknesses vary over wavelength; portions of the CO2 band and water vapor bands as well as ozone provide a direct radiative link between much of the stratosphere and much of the troposphere and surface.
2. Of course the upper stratosphere would be cooler if not for solar heating, mainly via ozone. The increase in temperature over height going into the upper stratosphere increases the emission to space from the center of the CO2 band, because the optical thickness at the center of the band is so great. Outside of that region, the source of LW emission to space is less concentrated into the upper atmosphere, and going outward from the center, first, less LW emission comes from the stratopshere as more comes from below, then less from the atmosphere as a whole as some can come from the surface when the optical thickness is not too large. If the upper stratosphere were not directly heated by the sun, the LW flux to space near 15 microns would be less, but it would not be zero.
3. Even if layers are not so much directly radiatively linked, they are indirectly linked by intervening layers. Changes in radiative heating/cooling at one level will cause temperature changes that cause changes in LW emission that cause changes in radiative heating at other levels.
… “the 15um band is definitely being emitted by CO2 in the Stratosphere, and the most likely sources of energy are absorption of sunlight by CO2 and Ozone in the Stratosphere, which is almost completely thermally and radiatively isolated from the Troposphere by the deep and very cold Tropopause.”
At/near 15 microns, the upper stratosphere is relatively insulated radiatively from the troposphere and surface by the lower stratosphere (perhaps this is what some have been refering to as the ‘tropopause layer’ – but the tropopause is just the base of that layer).
But, while it is true that accurate calculation of radiative fluxes as a function of temperature can be done for each wavelength independently of other wavelengths, the full effect of radiative fluxes on temperature is through fluxes over all wavelengths where optical properties allow, and changes in temperature likewise affect radiative emission over all wavelengths allowed by optical properties and the Planck function.
I think Patrick 027 and I are agreeing violently with each other. It is true that every frequency will be different. And I agree that some wing frequencies, unlikely to be absorbed or radiated at any level in the atmosphere, will make it through.
It’s just that for the CO2 frequencies, the main band is the 15um band – the 2.7um and 4.3um bands don’t figure much in the CO2 emission of energy to space.
The strongest (most energetic) emission bands are also those which are absorbed the strongest. At these frequencies half the emissions are absorbed in 25m at ground level, and in the last 0.2% of the atmosphere at the top of the atmosphere. There is zero chance that a photon at the peak frequencies going to Space is coming from anywhere near the Troposphere. It has to be from high in the Stratosphere.
Even at the wing frequencies (say Wavenumbers 600 through 700) the absorption is significant, and not much comes from below the Stratosphere.
I would like to see a calculation of the amount of power emitted to space by CO2 from the Troposphere, Tropopause and Stratosphere. At present my assessment is that the great majority must be emitted from the Stratosphere and that there is only very weak power being emitted from the Troposphere.
(continued from above)
Looking at the graphs above, in particular the outgoing LW radiation graphed relative to brightness temperature for 390 ppm:
Roughly estimating from the graph (so give or take a bit), it appears:
Within the 15 micron CO2 band, the brightness temperature hits bottom at about 640/cm to 700/cm
The brightness temperature starts differing from it’s maximum (heading into the CO2 band) around 570/cm and 760/cm
Going from 570/cm to 640/cm, and going from 760/cm to 700/cm, less and less LW radiation reaching space comes from the surface, and, at first, more and more from the troposphere, and then less from the troposphere and more from the stratosphere. At some point around 640/cm and 700/cm (though maybe not corresponding to the exact minima in brightness temperature), a majority comes from the stratosphere; and within that, a range where almost none comes from below the tropopause. But going into this range, what happens first is that most of the upward flux at the tropopause (from below) is absorbed within the stratosphere – more in the lower stratosphere, but with some still absorbed in the upper stratosphere. The point where nearly all fluxes across the tropopause are absorbed rather nearby is limited to smaller range of wavelengths.
There is a range of wavelengths in the center of the CO2 band where additional CO2 doesn’t cause much of a change, because the downward flux from above can’t be increased much more and the upward flux from below is coming from a rather short distance away. The radiative forcing caused by an increase in CO2 is caused by what happens outside that range. As more CO2 is added, this central portion where the tropopause level forcing is saturated expands, but so does the region where opacity has a significant effect – the wings expand outward, and so the intervals where adding yet more CO2 has an effect are still there, just shifted outward from the center. In effect, each doubling of CO2 tends to have a similar effect in widenning the band; the amount of widenning can be multiplied by the difference in fluxes between 1.where there is no opacity from CO2 and 2.where the CO2 effect is saturated, and that is approximately the radiative forcing (It’s not exact because the spectrum has some deviations from a triangular log(optical thickness) vs wavenumber or wavelength; and maybe a couple other things). The difference is reduced through a change in 1. by water vapor and clouds, but a difference between 1 and 2 remains. (Most of the water vapor and cloud overlap with CO2 only affects the upward flux at the tropopause; There is some significant, but not large, optical thickness from water vapor over some wavelengths within the stratosphere, but near the CO2 band, the little bit of stratospheric water vapor is nearly transparent).
Re https://chriscolose.wordpress.com/2010/02/18/greenhouse-effect-revisited/#comment-2248
That radiosonde info:
.11 g/kg (H2O/air) * 29/18 (g/mol air/ g/mol H2O) ~= 180 ppm (molar fraction) H2O/air
That’s really large for the stratosphere, isn’t it?
Do you think this radiosonde is representative of typical conditions?
How did the water get there? Was it methane oxydation? Something else?
—-
PS I was going to suggest that, depending on how the trajectories enter and exit the stratosphere, it would be conceivable that water vapor concentration could decrease with height above the tropopause for some range (because of the limited penetration of injections by severe thunderstorms and … mixing across jets?). Water vapor injected into the stratosphere into regions where the air is only a short distance and time away from reentry into the troposphere would tend to not reach higher up.
I thank Patrick 027 for his comments. I was also suprised.
See this site: http://weather.uwyo.edu/upperair/sounding.html
Have a look at: http://weather.uwyo.edu/cgi-bin/sounding?region=naconf&TYPE=TEXT%3ALIST&YEAR=2010&MONTH=04&FROM=2100&TO=2100&STNM=71867 which is from Manitoba,
and http://weather.uwyo.edu/cgi-bin/sounding?region=naconf&TYPE=TEXT%3ALIST&YEAR=2010&MONTH=04&FROM=2100&TO=2100&STNM=72582 which I think is from Nevada,
and http://weather.uwyo.edu/cgi-bin/sounding?region=naconf&TYPE=TEXT%3ALIST&YEAR=2010&MONTH=04&FROM=2100&TO=2100&STNM=91285 which I think is Hawaii .
All these show increasing water vapour in the Stratosphere.
There’s also the high artcic http://weather.uwyo.edu/cgi-bin/sounding?region=naconf&TYPE=TEXT%3ALIST&YEAR=2010&MONTH=04&FROM=2100&TO=2100&STNM=71924 somewhere near Elsmore Is, which is quite different.
As I said, I was quite taken aback. I can’t see any mechanism for water vapour to get into the high Stratosphere, nor can I explain the increasing amount as you go higher – there doesn’t seem to be a pathway from the Troposphere. The explanation might be an extraterrestial source or (and appositely of the moment!!) volcanic eruptions.
Some further observations/thoughts.
1. Many sites seem to show the same thing, increasing water vapour with height beyong the Tropopause.
2. Is it possible that this is due to leakage from the tropics?
Re Colin H2O in upper stratosphere –
Okay, I see what you mean. It looks weird to me. Systemic instrumental errors at low pressures?
Oxidation of CH4 is an option (not knowing if it would pan out, though) for persistent enhanced water vapor above the tropopause; otherwise it makes more sense for water vapor to remain steady (in g/kg) or decrease away from the tropopause into the stratosphere, so far as I know.
What becomes problematic is explaining how water vapor could accumulate to such high mixing ratios in the upper stratosphere without somewhere at sometime having those same mixing ratios appear in the lower stratosphere as the Brewer-Dobson circulation brings it back down at high latitudes. If the water vapor were patchy to begin with, it could be mixed horizontally before coming down; or maybe there is some vertical mixing (weaker than in the troposphere) that brings it down without maintaining the high concentrations … but this doesn’t fit what I thought. It’s puzzling to me. I’d want to know if there is a known bias or inaccuracy in the measurements at lower pressures.
I agree with Patrick 027, but it could also be a temperature bias in the instrumentation: higher temperature = higher reading.
Is Chris aware of errors in water vapour measurement at very high altitudes, or any scientific discussion of these results? I had a brief look on google, (avoiding everything talking about global warming, climate change and greenhouse effect) and the general consensus on water vapour in the stratosphere is that there isn’t any.
But there are the polar clouds (diamond dust?) and the noctiluminescent clouds.
… or could ozone be tricking the instrument (I don’t know the actual method of measurement used)?
Hi–first-time poster, hope this is in the right place.
In the earth energy budget diagrams, why is ALL of the back radiation (~320W m-2) absorbed by the earth’s surface? I realize that it’s at a different wavelength than the solar coming in, but some of that is reflected (30 out of ~200W m-2), so why shouldn’t some of the back radiation be reflected? Or is it just that they don’t or can’t measure reflected back-radiation?
And a related question, is the absorption the same for land as well as ocean?
Thanks in advance for any answers.
Response: Reflection of infrared radiation is generally an insignificant term on an Earthlike planet. In other atmospheres, such as Venus, you’d find that the reflection of infrared is a major term, however. The Kiehl, Trenberth, Fasullo type figures assume unit emissivity in the IR, which for most solid and liquid surfaces, is an excellent approximation, although in some cases it does introduce important error (emissivity is lowest in desert regions for example). The error at the surface could be on the order of about 5 W m-2 as discussed in their paper. Reflection in the visible, however, is very important. As for land vs. ocean surfaces, this depends on the surface (e.g., ice is very reflective, a parking lot is very absorbing), and for water also depends on the solar angle.– chris
Chris,
It’s too bad that one can’t remove the water vapor above 11 km as well as the N20 in Archer’s MODTRAN interface. You could then get rid of almost all the bumps and wiggles.
Don’t cirrus clouds have significant reflectivity of IR between 20 and 30 micrometers. I seem to remember that the lack of contrails over the North Pole immediately after 9/11/2001 had a measurable effect.
Response: Cirrus clouds mostly cause a warming effect through IR absorption. I don’t know where (if) their IR scattering influence ever becomes important or how things changed after 9/11. By the way, you can take water vapor out of the atmosphere although not above a particular altitude, although you can change the level at which the sensor is reading from and the upper levels are pretty dry– chris
I calculated spectra for mid-latitude summer with and without standard cirrus model clouds and subtracted the emission without from with. It mostly fits a 230 K Planck curve with an emissivity of 0.145 so there doesn’t look to be any significant reflection. There was a brief communication in Nature about an increase in the diurnal temperature range for 9/11-14 compared to the three days before and after.
Click to access jetcontrailsrecentresearch.pdf
Giant Fail. There is no discussion of heat capacity. Ask the “physicist” what the temperatures are of the various layers of the atmosphere as it just might surprise all of you. Temperature does not equal heat. Ask a plasma physicist There is no understanding about how heat capacity of the surface gas is impacted. Second, there is no discussion of the conductive nature of gases when contacting a surface. Note that there is more O2 and N2 and these gases can absorb thermal energy from the earth just by contacting it’s surface. Don’t believe me…take your vacuum thermos, fill it with hot water, and pump in some N2 to eliminate the vacuum. No more hot water 1 hour later. Why?? A nitrogen molecule can absorb all manner of thermal kinetic energy. If a CO2 molecule happens to get exicited with a 15 um photon, rare though they are, it can give it up with the same photon or give it up thermally. The post fails in other areas, it’s a known fact that the sun emits at 15um and all other wavelengths that are of interest. If more CO2 is preventing that energy from reaching the ground then the earth is cooler to start. Net change…zero!! In fact, if that energy is absorbed higher up in the atmosphere the earth will be cooler. Lastly, 5th grade science students were given a mirror, a lamp without a shade, and a white piece of paper. The mirror was held at a distance away, perpendicular to the plane between the paper and lamp. The paper is viewed without the mirror and with the mirror in place. The purpose of the mirror was, you guessed it, to simulate radiative forcing. If radiative forcing were real, the reflected light from the paper would get re-reflected off the mirror and back on to the paper and make it look brighter. Guess what didn’t happen. Remember this folks, a concensus of scientists said a rocket wouldn’t work in a vacuum. There’s nothing to push against….
Response: This is all confused. First, conduction is only really important at a very thin layer near the ground, and it’s an important component for surface exchange to the air right above it, but it’s not a component of the greenhouse effect. I seen no reason to squeeze a textbook of atmospheric physics into a blog post. Also, CO2 does absorb very small amounts of solar radiation but it’s extremely small relative to the upwelling longwave component;unless you are doing a detailed line by line calculation (as is done in Myhre et al 1998 for instance) it can be neglected. Once again this is more of a technical issue that needn’t be included in an introductory post. The rest of your post makes no sense whatsoever– chris
The reference to coal power plants just reemphasises the need for more and new nuclear plants to replace all the wasteful, polluting plants.
Unless I’m mistaken… “Greenhouse Gasses” work differently than glass; they also block heat rays from getting to the earth? If so, having more “greenhouse gasses” means that more heat rays reflect back into space at the same ratio of In vs Out… Therefore if all things remain relatively constant; It is simply an issue of the sun. When our sun is hotter the earth gets hotter, when the sun is colder we are colder. Of course big variables do exist; but I think they are primarily in outer space and well beyond our control.
Yes, greenhouse gases work differently than glass, but they (along with blankets/coats) have broadly similar effects in that they slow the rate at which heat can flow out from a warmer place (the climate system, a greenhouse, a body, a house, etc.) to a colder place or heat sink (space, outside air, surroundings), without affecting the rate of heat input (solar heating, metabolism, heat from people, furnaces and appliances (in a house), etc.)**. If the heat is supplied at the same rate and the rate of heat loss is changed, then heat will build up or become depleted until the flows balance (because the outflow of heat responds to the concentration of heat – a larger temperature gradient drives more heat flow through conduction and possibly convection (neither of which can usually carry significant amounts of heat to space from a planet, conduction being important only at the surface and within the land material (and in transfer of heat between air and particles within the air) and generally lumped together with convection in discussing climate, and global average convection is largely limited to the troposphere (so that the troposphere+surface tend to warm up or cool down together in response to radiant energy flows in and out of the tropopause), with deviations from that pattern which can be understood and predicted); larger temperature differences among emitters and absorbers of radiation drive a larger radiant heat flow (Planck response)). If the impedance of temperature-dependent heat outflow is not changed but the heat input is, then heat will again build up or become depleted until a balance is reached.
** Actual greenhouse gases and agents can also affect solar heating, but the two effects can be considered seperate processes; the effect on solar heating is not part of the ‘greenhouse effect’. CO2’s effect is mainly as a greenhouse gas; water vapor absorbs a signifcant amount of solar radiation, but this doesn’t have much effect on the energy balance of the surface+troposphere, except to the extent that it absorbs radiation that would otherwise have been reflected; water vapor’s greenhouse effect could be considered more important. Clouds’ effect on solar heating and greenhouse effect are generally both important.
Greenhouse gases and water clouds on Earth produce a greenhouse effect primarily by absorbing and emitting radiation; scattering (like reflection) is minor, though in other conditions (such as an extremely cold planet with dry-ice clouds) it can become important and a greenhouse effect could theoretically be based purely on scattering/reflection. On Earth, scattering and absorption are both important in affecting solar radiation.
Mississauga, you are to be commended for not simply accepting what others tell you but are prepared to think for yourself about the issue. Always check out the background and qualifications of self-appointed “experts”. Chris may have been taught more than you about this topic but he has not learnt everything yet. Even qualified research scientists have an awful lot to learn before being able to claim a proper understanding of the processes and drivers of global climates and there is significant disagreement between the different “experts”. A good example of this is the exchange last April involving two Adelaide University Professors, Barry Brook and Ian Plimer.
Brook, a staunch supporter of The (significant human-made global climate change) Hypothesis and chief scientific advisor to the last Australian Government, has acknowledged (Note 1) “There are a lot of uncertainties in science, and it is indeed likely that the current consensus on some points of climate science is wrong, or at least sufficiently uncertain that we don’t know anything much useful about processes or drivers”. Even the qualified experts disagree. Brooks made that comment in an article in which he was ridiculing the book “Heaven and Earth” in which Plimer challenges the validity of The Hypothesis.
Keep researching and developing your own understanding and don’t be fooled by claims of consensus, which has no relevance in science. A good starting point for you is The International Climate Science Coalition (Note 2) where you can find help from recognised professionals on most aspects of climate change.
edit— blabbering nonsense
“The effective height “H” above … moves higher as the opacity in increased in that region.”
Obviously, if that’s right, the total energy accumulated into the atmosphere would increase.
Comments.
1) The Earth’s atmosphere without GHGs, would be perfectly transparent to incoming and outgoing radiation, in thermal equilibrium with the surface and all at the same temperature Teff=255K. The total energy accumulated into the atmosphere would be E=255*Catm, where the last in the atmospheric thermal capacity.
2) The Standard Atmosphere Computations at
http://www.desktop.aero/appliedaero/appendices/stdatm.html
give for H=5475 m, P=50679 Pa (half a bar) and T=253 K. In other words, the under half atmospheric mass is warmer than 253K, whereas the remaining above half is colder than 253K. Then the total energy would be E=253*Catm, in practice the same of the isothermal atmosphere at point 1).
3) That means that GHG’s acting for millions of years hasn’t changed the atmosphere’s energy, the GHG’s effect is only to rotate the temperature gradient around the middle of atmosphere!!
That’s amazing, but then, what will happen to GW?
edit– Do you say to yourself “hey! Fresh prey” when someone who appears uncertain comes along, and then proceed to pounce with full force with the objective of confusing them? Please, do it elsewhere.
In your comments above you say that the Models are consistent with the observations. The Hadcut data show Global Temperatures increasing at 1.1C per century for the period from just after WW II when CO2 Concentrations began to rise at a reasonably steady rate through 2010. This is considerably different than the 1.5 to 4.5 C from the models, particularly when one considers that the lower end of the range is for a slowing rate of CO2 emissions.
Response: The numbers you quote sound much more like the expected equilibrium sensitivity for a doubling of CO2, not the effects at the year 2010 (which is neither in equilibrium nor at a doubling of pre-industrial CO2)– chris.